lesson 4 problem solving practice dividing rational numbers

• → Curriculum
• → 7th Grade
• → Unit 1: Rational Numbers

Dividing Rational Numbers Lesson Plan

Get the lesson materials.

Dividing Rational Numbers Fractions Decimals Guided Notes Sketch & Doodles

Ever wondered how to teach dividing rational numbers, including fractions, integers, and decimals, in an engaging way to your middle school students?

In this lesson plan, students will learn about dividing rational numbers and their real-life applications. Through artistic and interactive guided notes, check for understanding questions, a color by code activity, and a maze worksheet, students will gain a comprehensive understanding of dividing rational numbers.

The lesson culminates with a real-life example that explores how dividing rational numbers can be applied to splitting a bill at a restaurant.

• Standards : CCSS 7.NS.A.2 , CCSS 7.NS.A.2.a , CCSS 7.NS.A.2.c
• Topics : Integers & Rational Numbers , Fractions , Decimals
• Grade : 7th Grade
• Type : Lesson Plans

Learning Objectives

After this lesson, students will be able to:

Divide rational numbers, including fractions, integers, and decimals

Solve division problems involving positive and negative rational numbers

Apply division of rational numbers to real-life situations

Prerequisites

Before this lesson, students should be familiar with:

Basic operations with rational numbers (adding, subtracting, and multiplying)

Basic understanding of fractions and decimals

Knowledge of how to determine the greatest common factor (GCF) and least common multiple (LCM) of numbers

Colored pencils or markers

Dividing Rational Numbers Fractions Decimals Guided Notes

Key Vocabulary

Rational numbers

Introduction

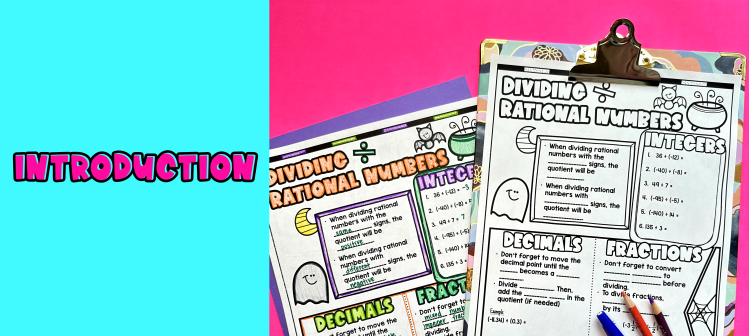
As a hook, ask students why dividing rational numbers, including fractions, integers, and decimals, is important in real life. Refer to the real-life math application on the last page of the guided notes as well as the FAQs below for ideas.

Use the guided notes to introduce the concept of dividing rational numbers. Walk through the key points of the topic, including the steps and techniques involved in dividing rational numbers. Refer to the FAQ below for a walk through on this, as well as ideas on how to respond to common student questions.

Check for Understanding : Have students walk through the "You Try!" section of the guided notes. Call on students to talk through their answers, potentially on the whiteboard or projector. Based on student responses, reteach concepts that students need extra help with.

Based on student responses, reteach concepts that students need extra help with. If your class has a wide range of proficiency levels, you can pull out students for reteaching, and have more advanced students begin work on the practice exercises.

Have students practice dividing rational numbers including fractions, integers, and decimals using the color by code activity included in the resource. Walk around the classroom to answer any student questions and provide assistance as needed.

Fast finishers can work on the maze activity for extra practice. You can assign these activities as homework for the remainder of the class.

Real-Life Application

Bring the class back together, and introduce the concept of rational number division applied to splitting a bill with friends. Refer to the FAQ for more real life applications that you can use for the discussion!

Additional Self-Checking Digital Practice

If you’re looking for digital practice for dividing rational numbers, try my Pixel Art activities in Google Sheets. Every answer is automatically checked, and correct answers unlock parts of a mystery picture. It’s incredibly fun, and a powerful tool for differentiation.

Here's an activity to try:

Multiplying & Dividing Rational Numbers Digital Pixel Art

Additional Print Practice

A fun, no-prep way to practice dividing rational numbers is Doodle Math — they’re a fresh take on color by number or color by code. It includes multiple levels of practice, perfect for a review day or sub plan.

Multiplying & Dividing Rational Numbers | Doodle Math: Twist on Color by Number

What is dividing rational numbers? Open

Dividing rational numbers involves dividing numbers that can be expressed as fractions or decimals. It is the process of finding how many times one number can be evenly divided by another number.

How do you divide fractions? Open

To divide fractions, you multiply the first fraction by the reciprocal (flipped) form of the second fraction. This can be done by multiplying the numerators together and the denominators together. Simplify the resulting fraction if possible.

How do you divide decimals? Open

Dividing decimals is similar to dividing whole numbers. Use long division to divide the decimal dividend by the decimal divisor. Place the decimal point in the quotient directly above the decimal point in the dividend.

Can you divide positive and negative rational numbers? Open

Yes, you can divide positive and negative rational numbers. The rules for dividing positive and negative numbers are the same as for multiplying them. The result of the division will have a positive quotient if both numbers have the same sign, and a negative quotient if the numbers have different signs.

What is the difference between dividing fractions and dividing decimals? Open

The main difference is in the representation of the numbers. Dividing fractions involves dividing numbers expressed as fractions, while dividing decimals involves dividing numbers expressed as decimal numbers. The processes and calculations are similar, but the final answers may be in different forms.

How can dividing rational numbers be applied in real life? Open

Dividing rational numbers is commonly used in real-life situations such as dividing the bill for a pizza among friends, calculating the cost per unit of a product, or determining the average speed of a moving object. It helps in solving problems that involve sharing, distributing, or comparing quantities.

Are there any tips or tricks for dividing rational numbers? Open

One tip for dividing rational numbers is to always simplify the fraction before dividing. This makes the calculation easier and reduces the chances of errors. Additionally, keeping track of the signs (+/-) and placing the decimal point correctly when dividing decimals will help in obtaining accurate results.

What are some common mistakes to avoid when dividing rational numbers? Open

Common mistakes to avoid when dividing rational numbers include forgetting to simplify the fraction, reversing the order of the fractions when finding the reciprocal, misplacing the decimal point when dividing decimals, and forgetting to consider the signs of the numbers being divided.

Are there any resources available to practice dividing rational numbers? Open

Yes, there are various resources available for practicing dividing rational numbers. This lesson plan includes guided notes, practice worksheets, color by code activities, and a real-life math application.

Want more ideas and freebies?

Get my free resource library with digital & print activities—plus tips over email.

Multiplying and dividing rational numbers

In this lesson we will learn about multiplication and division of rational numbers.

How to multiply rational numbers

The rules for multiplying integers are also true for rational numbers. In other words, to multiply rational numbers, you must be able to multiply integers .

It is also necessary to know the basic laws of multiplication such as the commutative law of multiplication, the associative law of multiplication, the distributive law of multiplication, and the law of multiplication by zero.

This is multiplication of rational numbers with different signs. To multiply rational numbers with different signs, multiply their modules and put minus sign in front of the result.

To clearly see that we are dealing with numbers with different signs, put each rational number in parentheses along with its signs

The short solution is as follows:

This is a multiplication of rational numbers with different signs. Multiply the modules of these numbers and put minus in front of the answer:

The solution for this example can be written in a shorter form:

This is multiplication of negative rational numbers. To multiply negative rational numbers, multiply their modules and put plus sign in front of the answer

This is a multiplication of negative rational numbers. Multiply the modules of these numbers and put plus in front of the answer

This is a multiplication of rational numbers with different signs. Multiply the modules of these numbers and put minus in front of the answer

A short solution would look much easier:

We obtained the multiplication of rational numbers with different signs. Multiply the modules of these numbers and put minus in front of the answer. You can skip the entry with modules, so as not to overload the expression

The solution for this example can be written in shorter form

An expression consists of several factors. According to the associative law of multiplication, if an expression consists of several factors, then the product will not depend on the order of operations. This allows us to calculate the expression in any order.

Second act:

Let's convert the mixed numbers into improper fraction:

We obtained the multiplication of negative rational numbers. Multiply the modules of these numbers and put plus in front of the answer. You can skip the entry with modules, so as not to overload the expression

Example 10. Find the value of the expression

Therefore let us calculate this expression from left to right in the order of the factors. Let us omit the entry with modules in order not to overload the expression

Fourth act:

Recall the law of multiplication by zero. This law states that a product is zero if at least one of the factors is zero.

A product is zero if at least one of the factors is zero.

According to the order of operations , if an expression contains addition and multiplication, then multiplication should be performed first. Therefore, in the resulting new expression, we bracket the fractions that should be multiplied. This way we can clearly see which actions to perform first, and which later:

Next, we calculate the expression by actions. First, we calculate the expressions in parentheses and add the results

The solution for this example can be written in a much shorter form. It would look as follows:

You can see that this example could be solved even in your mind. Therefore, you should develop the skill of analyzing the expression before you start solving it. It is likely that you can solve it in your head and save a lot of time and nerves. And on tests and exams, as you know, time is very expensive.

Example 14. Find the value of the expression -4.2 × 3.2

Notice how the modules of rational numbers were multiplied. In this case, in order to multiply modules of rational numbers, you had to be able to multiply decimals .

Example 15. Find the value of the expression -0.15 × 4

Notice how the modules of rational numbers were multiplied. In this case, to multiply modules of rational numbers, you had to be able to multiply a decimal and an integer .

Example 16. Find the value of the expression -4.2 × (-7.5)

How to divide rational numbers

Division of rational numbers is reduced to the multiplication of the same numbers. To do this, the first fraction is multiplied by the inverse of the second fraction. Then the rules of multiplication of rational numbers are applied.

We got the multiplication of rational numbers with different signs. And we already know how to calculate such expressions. To do this we need to multiply the modules of the given rational numbers and put minus in front of the obtained answer.

Let's finish this example to the end. You can skip the entry with modules to avoid overloading the expression

The detailed solution is as follows:

The short solution can be written as follows:

We obtained the multiplication of negative rational numbers. Let us perform this multiplication. You can skip the entry with modules in order not to overload the expression:

Multiply the first fraction by the number inverse of 4.

Multiply the first fraction by the number inverse of -3

Example 6: Find the value of the expression -14.4 : 1.8

This is the division of rational numbers with different signs. To calculate this expression, divide the modulus of the divisor by the modulus of the divisor and put minus sign in front of the answer

Notice how the modulus of the divisor was divided by the modulus of the divisor. In this case, we needed to be able to divide a decimal by another decimal .

If there is no desire to work with decimals (which is often the case), these decimals can be converted into mixed numbers , then you can convert these mixed numbers into improper fraction, and then deal directly with division.

Calculate the previous expression -14.4 : 1.8 this way. Convert the decimal fractions into mixed numbers:

Now convert these mixed numbers into improper fraction:

Example 8. Find the value of the expression -7.2 : (-0.6)

This is division of negative rational numbers. To do this division, multiply the first fraction by the inverse of the second fraction.

Move the comma in both fractions one digit to the right to obtain division

Complex fraction

In the first case, the division sign is a colon and the expression is written on one line. In the second case, the division of fractions is written with a fractional dash. The result is a fraction, which is called a complex fraction .

When encountering such complex fractions, you must apply the same rules for dividing regular fractions. The first fraction must be multiplied by the inversed second fraction.

It is extremely inconvenient to use such fractions in the solution, so you can write them in an understandable form, using a colon instead of a fractional line as the division sign.

After determining the main fractional line, you can easily figure out where the first fraction is and where the second fraction is:

And then you can use the method of dividing fractions - multiply the first fraction by the inversed second one.

Despite the fact that complex fractions are awkward to work with, we will encounter them very often, especially when studying higher mathematics.

Naturally, it takes extra time and space to convert complex fractions into an understandable form. So we can use a faster method. This method is convenient and yields a ready-made expression with the first fraction multiplied by the inversed second one.

This method is implemented as follows:

To avoid mistakes when using this method, you can follow the following rule:

From the first to the fourth. From the second to the third.

The rule refers to the "levels". The figure from the first "level" should be raised to the fourth "level". And the figure from the second "level" should be raised to the third "level".

There is only the first, second and fourth "level". The third "level" is missing. But we do not deviate from the basic scheme: we raise the figure from the first  "level" to the fourth "level". And since the third "level" is missing, we leave the figure on the second "level" as it is

There is only the second, third and fourth "level". The first "level" is missing. Since the first "level" is missing, we don't need to go up to the fourth "level", but we can raise the number from the second "level" to the third:

Using variables

If an expression is complicated and you think it will confuse you as you solve a problem, you can put part of it into a variable, and then work with that variable.

Mathematicians often do this. They break a complex problem into easier subproblems and solve them. Then they assemble the solved subtasks into a single whole. This is a creative process, and it takes years of hard practice to learn.

The use of variables is justified when working with complex fractions. For example:

So there is a fractional expression in the numerator and a fractional expression in the denominator. In other words, we are again faced with the complex fraction that we dislike so much.

But in mathematics, in such a case, it is customary to name variables with large Latin letters. Let us not break this tradition, and denote the first expression by the capital Latin letter - A

Let us find the value of variable A

Let's find the value of variable B

We got a complex fraction in which we can use the scheme " from the first to the fourth, from the second to the third ", that is, the figure that is on the first "level" to raise to the fourth "level", and the figure that is on the second "level" to raise to the third "level". Further calculation is not too difficult:

Of course, we looked at the simplest example, but our goal was to see how we could use variables to make things easier for ourselves, so that we could minimize errors.

Note also that the solution to this example can be written without using variables. It would look like this

This solution is faster and shorter, and in this case it would be better to write it that way, but if an expression turns out to be complex, consisting of several parameters, parentheses, roots, and powers, it is advisable to calculate it in several steps, putting some of its expressions into variables.

Video lesson

• Adding and Subtracting Rational Numbers
• More information about fractions

Add comment

Name (required)

E-mail (required, but will not display)

Notify me of follow-up comments

• You are here:  
• Home >>
• 37. Multiplying and dividing rational numbers
• 2. Basic operations
• 3. Expressions
• 4. Substitutions in expressions
• 5. Place value for beginners
• 6. Multiplication
• 7. Division
• 8. Order of operations
• Basic Laws of Math
• 9. Basic Laws of Math
• 10. Divisors and multiples
• 11. GCD and LCM
• 12. Fractions
• 13. Actions with fractions
• 14. Mixed numbers
• 15. Comparing Fractions
• 16. Units of Measurement
• 17. Ways to use fractions
• 18. Decimals
• 19. Operations with decimals
• 20. Using decimals
• 21. Rounding numbers
• 22. Repeating Decimals
• 23. Unit conversion
• Ratios & Proportion
• 25. Proportion
• 26. Distance, speed, time
• 27. Direct and inverse proportions
• 28. What are percentages?
• Additional topic & Number line
• 29. Negative numbers
• 30. Absolute value or modulus
• 31. What is a set?
• 32. Adding and subtracting integers
• 33. Multiplication and division of integers
• Rational numbers
• 34. Rational numbers
• 35. Comparing rational numbers
• 36. Adding and subtracting rational numbers
• 38. More information about fractions
• 39. Algebraic expressions
• 40. Taking out a common factor
• 41. Expanding brackets
• 42. Simple math problems
• 43. Tasks on fractions
• 44. Percent worksheets for practice
• 45. Speed, distance, time tasks
• 46. Productivity problem
• 47. Statistics in Math
• 48. Basic theory of equation
• 49. Problem solving with equations
• 50. Proportions: exercises
• 51. Systems of linear equations
• 52. Introduction to Inequalities
• 53. Systems of linear inequalities with one variable
• 54. Set Operations | Union | Intersection
• 55. Power with a natural exponent
• 56. Power with integer exponent
• 57. Perimeter, area and volume
• 58. Monomials
• 59. Polynomials
• 60. Reduced multiplication formulas
• 61. Polynomial decomposition
• 62. Dividing polynomials
• 63. Identical transformations of polynomials
• 64. Square root
• 65. Algorithm for extracting the square root
• 66. Quadratic equation
• 67. A quadratic equation with an even second coefficient
• 68. Viette's Theorem
• 69. Factoring a trinomial using decomposition
• 70. More about modules
• 71. Absolute value equations
• 72. Solving equations with module by method of intervals
• 73. Inequalities with module
• 74. Solving inequalities with module by method intervals
• 75. Square root from both parts of an equation
• Functions and graphs
• 76. What is a function?
• 77. Linear function and its graph
• 78. Power function
• 79. Direct Proportion and its Graph
• 80. Function y=x² and its graph
• 81. Function y = √x its properties and graph
• 82. Function y=k*x² its properties and graph
• 83. Function y=k/x its properties and graph
• 84. Graphical solution of equations and inequalities
• coming soon... (new lessons every month)

• Français
• Preparatory

Lesson Video: Dividing Rational Numbers Mathematics • First Year of Preparatory School

Join nagwa classes.

Attend live Mathematics sessions on Nagwa Classes to learn more about this topic from an expert teacher!

• Remaining Seats: 10

In this video, we will learn how to divide rational numbers, including fractions and decimals.

Video Transcript

In this lesson, what we’ll be looking at is dividing rational numbers. And this will include fractions and decimals. So by the end of the lesson, what we should be able to do is divide a rational decimal by a rational decimal, divide a fraction by a fraction, divide rational numbers in various different forms, and, finally, solve word problems involving the division of rational numbers.

But before we start to do any of that, what is a rational number? Well, in fact, a rational number is a real number that can be written as 𝑎 over 𝑏, where 𝑎 and 𝑏 are integers and 𝑏 is not equal to zero. Another way of saying this is that it is any number that can be represented as the ratio between two integers. So what we have here are some examples. So first of all, we’ve got two-fifths which we can see is written in the form 𝑎 over 𝑏. Well, then we have 0.3 recurring. But we think, “hold on! This isn’t written as 𝑎 over 𝑏. So how come this is a rational number?” Well, 0.3 recurring can be written as one over three or one-third. So it is also worth noting at this point that, in fact, any recurring decimal is in fact a rational number. Then we have three.

But, again, we’re thinking, well, that’s not in the form 𝑎 over 𝑏. Well, in fact, it could be written as three over one or even nine over three; they would both give us three. Then we have another recurring decimal, which is 0.142857 recurring. This time, I’ve just put this in here just to show there’s a different way of showing recurring here. And that’s with a straight line, not just a dot. We could have a dot over the one and a dot over the seven to mean the same thing. And as we said before, all recurring decimals are rational numbers. And this one is the same as one over seven or one-seventh. Then, finally, for our examples, we have 0.125, which you might already know is an eighth. And it’s also worth pointing out here is that this is something called a terminating decimal. And any terminating decimal is also a rational number.

So therefore, what we can also surmise is that the converse of this, anything that doesn’t satisfy this rule, is not going to be a rational number. It’s going to be an irrational number. Okay, great. So we’ve looked at what rational numbers are. So now what we’re going to do is move on to our questions.

Evaluate 0.8 divided by 0.4.

So what we have here are two terminating decimals, and we’re going to divide them. And we have a couple of methods to do this. So what we’re gonna do is have a look at both of them. So for method one, what we’re gonna do is we’re gonna multiply both of our decimals. And we’re gonna multiply them both by 10. And that’s because what we’re gonna do is make it so, in fact, we’re not dividing decimals at all. We’re gonna be dividing units. So if we multiply 0.8 and 0.4 by 10, what’s gonna happen is that each of the numbers is going to move one place value to the left. So what we’re gonna get is eight divided by four. And we can do this because we’ve done it to both terms. So therefore, it’s going to give us the same result. Well, this is nice and straightforward. And that’s because eight divided by four is equal to two. So therefore, we can say that 0.8 divided by 0.4 is two.

So now we’re gonna take a look at method two. And for method two, what we’re going to do is convert both of our decimals to fractions. And we can do that because they’re both terminating decimals. And we know that terminating decimals are rational numbers, so therefore can be converted to a fraction with an integer as the numerator and an integer as the denominator. Well, if we start with 0.8, what this means is eight-tenths. Well, in turn, we can simplify eight-tenths by dividing the numerator and denominator by two, which will give us four-fifths. Then we have 0.4, which is gonna be equal to four-tenths, which again we can simplify by dividing the numerator and denominator by two to give us two-fifths.

Okay, great. We now got our two fractions. So we now have four-fifths divided by two-fifths. And we’ve got a method for dividing fractions. And what we can do is use our memory aid to remind us how to do that. And that is KCF, which is keep it, change it, flip it. And this means we keep the first fraction the same, we change the sign from a divide to a multiply, and we flip the second fraction. And it’s worth noting that if we flip a fraction, this is in fact the reciprocal of that fraction. And then if we multiply two fractions, all we do is multiply the numerators and multiply the denominators. So this is gonna be equal to 20 over 10, which once again gives us an answer of two.

Now this is quite straightforward because we’re multiplying two easy fractions. However, there is a quick tip, which can be useful. If you’re ever multiplying fractions, have a look at the numerators and denominators and see if there’s a common factor. So here we can see that five is a common factor. So if we divide both the numerator and denominator by five, we’re just left with four multiplied by one over one multiplied by two, which is four over two, which again would’ve given us two.

So that was our first example. So now what we’re gonna do is have a look at an example where we’re going to evaluate an expression using multiplication and division of rational numbers. And all of these are gonna be in fractional form.

Evaluate three-quarters multiplied by negative two over three divided by a fifth giving the answer in its simplest form.

So to help us evaluate this expression, what we’re going to use is PEMDAS. And what PEMDAS is, is a way of remembering the order of operations. So here it says that the P — we’re gonna deal with the parentheses first, then exponents, multiplication, division, addition, then subtraction. So we can see that, in our expression, what we have are parentheses, so we’ll deal with these first. So what we have is three-quarters multiplied by negative two over three. So to multiply fractions, what we do is multiply numerators then multiply denominators. So this is gonna give us negative six over 12. Well, we can simplify this by dividing both the numerator and the denominator by six. So we’re gonna get negative one over two. So now we’ve dealt with our parentheses. So what we can do is put this value back into our expression.

Well, next we have a division. And that division is negative one over two, which is the result of the parentheses calculation, divided by one over five or one-fifth. Now, to help us remember what we’re gonna do with the division of fractions, we use our memory aid KCF, keep it, change it, flip it. So we keep the first fraction the same. We change our divide to a multiply. And we flip our second fraction. So we get negative one over two multiplied by five over one. So then what we do is multiply our numerators and denominators. So we can say that if we evaluate three-quarters multiplied by negative two over three divided by a fifth, we’re gonna get negative five over two.

So what we had a look at here was a problem involving fractions. What we’re gonna have a look at now is a problem that involves recurring decimals and the modulus or absolute value.

Evaluate 0.8 recurring divided by the modulus or absolute value of negative five over four giving the answer in its simplest form.

So in this question, the first thing we’re gonna have a look at is the recurring decimal. So we got 0.8 recurring. Now, this is, in fact, a rational number. And it’s a rational number because we can represent it as a fraction with an integer as the numerator and an integer as the denominator. And this is something we know about all recurring decimals. So to change this into a fraction, what we’re gonna do is let 𝑥 be equal to 0.8 recurring. And then what we’re gonna do is multiply 𝑥 by 10 to give us 10𝑥 and multiply 0.8 recurring by 10 to give us 8.8 recurring. So we now know that 10𝑥 is equal to 8.8 recurring.

So you might have thought, “Well, why have we just done that?” But it’s actually rather clever because what we’re gonna do now is eliminate the recurring part of our decimal. So let’s label them equation one and equation two. So what we can do is we can subtract equation one from equation two. So when we do that, what we’re gonna get on the left-hand side is nine 𝑥, cause we’ve got 10𝑥 minus 𝑥 which is nine 𝑥. And then on the right-hand side, we’re just gonna have eight, and that’s because if we have 8.8 recurring minus 0.8 recurring, the recurring parts cancel each other out and we’re left with just eight. So then what we’re gonna do is just divide through by nine. And what we get is 𝑥 is equal to eight over nine or eight-ninths. And as 𝑥 is equal to 0.8 recurring, we can say that 0.8 recurring is equal to eight over nine or eight-ninths. And this is a fraction with an integer numerator and an integer denominator.

Okay, great. So we converted that into a fraction. Well, now what about our absolute value or modulus of negative five over four? Well, these vertical lines mean the absolute value or modulus. And what this means is that we’re only interested in the positive value because what the absolute value or modulus means is the distance from zero or magnitude of a value. So therefore, we’re not interested in the negative part. So therefore, what we can do is just write our modulus or absolute value of negative five over four as five over four. So now what our calculation has become is eight over nine or eight-ninths divided by five over four. So now what we’re gonna do is divide our fractions.

And to remember how to do that, we can use our trusty memory aid, KCF: keep it, change it, flip it. So, to use this, we keep the first fraction the same. Then we change the sign from a divide to a multiply. And now we flip the second fraction. So we’ve now got eight over nine multiplied by four over five. So then if we multiply our numerators and denominators, we’re gonna get 32 over 45. And we can see this can’t be canceled down any further. So it is, in fact, in its simplest form. So then we can say the answer is 32 over 45.

Okay, great. So we’ve looked at a number of different skills so far, but what we’re gonna have look at now is to see if we can use these skills to solve problems. And what we’re gonna try and do is find our missing value.

The product of two rational numbers is negative 16 over nine. If one of the numbers is negative four over three, find the other number.

So the first thing we’re gonna do is look at a couple of key terms. So we’ve got product, which means multiply. So if we find the product of two numbers, that means we’re multiplying them together. And then we’re also looking at the term, rational. And what this means is a number that can be written as a fraction with an integer as the numerator and an integer as the denominator, which is gonna help us when we’re gonna try and find the number that we’re looking for. So taking the information we’ve got from the question, what we can do is write it down. And we’ve got negative four over three multiplied by 𝑎 over 𝑏 equals negative 16 over nine. And it’s this 𝑎 over 𝑏 that we’re trying to find.

Well, there are, in fact, a couple of ways we could solve this. So we’re gonna have a look at both of those. So first of all, what we could do is divide both sides by negative four over three. So when we do that, we’ll have 𝑎 over 𝑏 equals negative 16 over nine divided by negative four over three. So then what we can do is divide our fractions. And to do that, we can use our memory aid, KCF — keep it, change it, flip it — which is gonna give us 𝑎 over 𝑏 is equal to negative 16 over nine multiplied by negative three over four. So now, before we multiply, what we can do is divide through by any common factors. Well, first of all, we can divide numerators and denominators by four and then by three.

So now what we’ve got is negative four over three multiplied by negative one over one. Well, a negative multiplied by a negative is a positive. So therefore, what we’re gonna get is 𝑎 over 𝑏 is equal to four over three. So therefore, we’ve found our missing number. And what we can do is check this by using the alternate method. And the alternate method is equating the numerators and denominators. Well, as we know, we’ve got negative four over three in the left-hand side and the result is negative 16 over nine. We know that a negative has to be multiplied by a positive to give us a negative result. So therefore, we know that 𝑎 over 𝑏 will be positive. So we could ignore the signs when we’re gonna equate the numerators and denominators.

Well, if you equate the numerators, we’ve got four 𝑎 cause four multiplied by 𝑎 is equal to 16. So therefore, 𝑎 will be equal to four. So then if we equate the denominators, we’re gonna get three 𝑏 is equal to nine. So 𝑏 is equal to three. So therefore, 𝑎 over 𝑏 is gonna be equal to four over three, which is what we’ve got with the first method.

Okay, great. So we’ve just looked at a problem where we had to find a missing value. So for our final example, we’re gonna take a look at a worded problem, so a problem where we’re gonna use these skills in context.

Noah constructs three-quarters of a wall in one and two-thirds days. How many days will he need to construct the wall?

So what we’re gonna do is use a diagram to help us visualize the problem. So what we can see is it takes one and two-thirds days to build three-quarters of the wall. So therefore, if we want to find out how long it takes to build one-quarter of the wall, what we can do is divide one and two-thirds by three. Well, to complete this calculation, what we want to do is convert one and two-thirds, a mixed number, into a top heavy or improper fraction. So to do that, what we do is we see that there are three-thirds in a whole one add two gives us five over three. So it’s one multiplied by three add two over three. So we’ve got five-thirds divided by three.

Well, if we want to divide five-thirds by three, we can think of this as five-thirds divided by three over one. Well then what we can use is our memory aid for dividing by a fraction, which is keep it, change it, flip it, KCF, which will give us five-thirds multiplied by one over three, cause we keep the first fraction, change the sign, flip the second fraction. So therefore, what we’ve got is the time taken for one-quarter of the wall to be built is five over nine or five-ninths because five multiplied by one is five and three multiplied by three is nine.

So now what’s the next stage? Now what do we need to do? Well, we can see that the whole wall is four-quarters. So therefore, we need to multiply the time taken for a quarter of the wall to be built by four. So this means five-ninths multiplied by four. Well, to help us think what we’ll do with this calculation, we can think of four as four over one. So then we’re gonna do five multiplied by four over nine, which is equal to 20 over nine. Well, this is an improper fraction. So what we could do now is convert this back into a mixed number. Well, to do that, what we do is we see how many nines go into 20, which is two with a remainder of two. So therefore, we know that it takes two and two-ninths days to construct the wall.

In fact, it is worth noting that we could’ve completed this question with one calculation. And that would’ve been one and two-thirds multiplied by four over three because if we divide by three and multiply by four, it’s the same as multiplying by four over three. And it could also have been solved by the calculation one and two-thirds divided by three over four because if we go backwards from keep it, change it, flip it, we could see that one and two-thirds divided by three over four is the same as one and two-thirds multiplied by four over three.

So we’ve taken a look at a number of examples and covered the key objectives for the lesson, so now let’s take a look at the key points. So the first key point is that a rational number is in fact a real number that can be written as 𝑎 over 𝑏, where 𝑎 and 𝑏 are integers and 𝑏 is not equal to zero. So therefore, it can be written as a fraction. Another way we can think of that is that a rational number is a number that can be represented as the ratio of two integers. And we also know that both recurring decimals and terminating decimals are also rational numbers because these can be written as fractions.

For example, on the left, we have our recurring decimal, which can be written as a seventh. And on the right, we have a terminating decimal which could be written as an eighth. And for our final key point, if we’re gonna divide two fractions, so 𝑎 over 𝑏 divided by 𝑐 over 𝑑, then this is equal to 𝑎 over 𝑏 multiplied by 𝑑 over 𝑐. So you multiply it by the reciprocal with the second fraction. And we have a memory aid to help us remember this. And that is KCF: keep it, change it, flip it. Keep the first fraction the same, change the sign from a divide to a multiply, and flip the second fraction.

Attend live sessions on Nagwa Classes to boost your learning with guidance and advice from an expert teacher!

• Interactive Sessions
• Chat & Messaging
• Realistic Exam Questions

Nagwa uses cookies to ensure you get the best experience on our website. Learn more about our Privacy Policy

Curriculum  /  Math  /  7th Grade  /  Unit 2: Operations with Rational Numbers  /  Lesson 4

Operations with Rational Numbers

Lesson 4 of 18

Criteria for Success

Tips for teachers, anchor problems, problem set, target task, additional practice.

Model the addition of integers using a number line.

Common Core Standards

Core standards.

The core standards covered in this lesson

The Number System

7.NS.A.1.B — Understand p + q as the number located a distance |q| from p, in the positive or negative direction depending on whether q is positive or negative. Show that a number and its opposite have a sum of 0 (are additive inverses). Interpret sums of rational numbers by describing real-world contexts.

7.NS.A.1.D — Apply properties of operations as strategies to add and subtract rational numbers.

Foundational Standards

The foundational standards covered in this lesson

6.NS.C.6 — Understand a rational number as a point on the number line. Extend number line diagrams and coordinate axes familiar from previous grades to represent points on the line and in the plane with negative number coordinates.

6.NS.C.7.C — Understand the absolute value of a rational number as its distance from 0 on the number line; interpret absolute value as magnitude for a positive or negative quantity in a real-world situation. For example, for an account balance of -30 dollars, write |-30| = 30 to describe the size of the debt in dollars.

The essential concepts students need to demonstrate or understand to achieve the lesson objective

• Model addition on a number line using concepts of absolute value and the sign of the number to determine magnitude and direction.
• Determine that the commutative property of addition holds for the addition of integers.
• Represent integer addition problems on a number line.
• Write integer addition problems from models on a number line. 
• Model with mathematics using number lines, equations, and descriptions to show the relationships between the representations (MP.4).

Suggestions for teachers to help them teach this lesson

• In the next three lessons, students begin to operate with signed numbers. In this lesson, they start by building a conceptual understanding of the operation of addition using a number line and familiar contexts. Then in later lessons, students determine efficient ways to add rational numbers without the use of a number line. 
• This lesson only uses integers to allow for students to focus on the concepts and not calculations with fractions or decimals. Other rational numbers will be used in Lessons 5 and 6. 

Lesson Materials

• Laminated number line (1 per student)
• Dry erase marker (1 per student)
• Game piece or token (1 per student)

Unlock features to optimize your prep time, plan engaging lessons, and monitor student progress.

Problems designed to teach key points of the lesson and guiding questions to help draw out student understanding

25-30 minutes

The number line below represents the road that Joshua lives on, with his home located at point 0. The numbers on the number line represent the number of miles from Joshua’s house, either east or west. Throughout the week, Joshua goes on trips and errands along this road. 

For each day described in the chart, model Joshua’s trip and determine where on the number line he ends up each day. Write an addition equation to represent it.

Guiding Questions

Is $${-7+4}$$ equivalent to $${4+(-7)}$$ ? 

Show the sum for each expression on a number line to justify your answer.

In part (a), model the addition problem on the number line to find the sum. In part (b), write an addition equation to represent what is shown on the number line.

a.       $${5+(-4)+(-3)}$$

A set of suggested resources or problem types that teachers can turn into a problem set

15-20 minutes

Give your students more opportunities to practice the skills in this lesson with a downloadable problem set aligned to the daily objective.

A task that represents the peak thinking of the lesson - mastery will indicate whether or not objective was achieved

5-10 minutes

Represent each addition problem in parts A through C on a number line and find each sum.

a.    $${-9+5}$$

b.    $${8+(-7)}$$

c.    $${-3+(-6)}$$

d.   Choose one problem from A through C and write a real-world situation that could be modeled by the problem.

Student Response

The following resources include problems and activities aligned to the objective of the lesson that can be used for additional practice or to create your own problem set.

• Include problems where students go between addition equations and number line models (e.g., given one, write the other).
• Include error analysis problems where addition equations have been incorrectly written to represent a number line diagram (see notes in Anchor Problem #3).
• Illustrative Mathematics Distances on the Number Line 2
• EngageNY Mathematics Grade 7 Mathematics > Module 2 > Topic A > Lesson 3 — Problem Set #1 only
• EngageNY Mathematics Grade 7 Mathematics > Module 2 > Topic A > Lesson 2 — Examples, Exercises, and Problem Set. (Note, some problems reference the Integer Game. If this game is not being used in class, the problems can be modified to remove the reference.)

Topic A: Adding and Subtracting Rational Numbers

Represent rational numbers on the number line. Define opposites, absolute value, and rational numbers.

Compare and order rational numbers. Write and interpret inequalities to describe the order of rational numbers.

Describe situations in which opposite quantities combine to make zero.

7.NS.A.1.B 7.NS.A.1.D

Determine efficient ways to add rational numbers with and without the number line.

Efficiently add and reason about sums of rational numbers.

Understand subtraction as addition of the opposite value (or additive inverse).

7.NS.A.1.C 7.NS.A.1.D

Find and represent the distance between two rational numbers as the absolute value of their difference.

Subtract rational numbers with and without the number line.

Add and subtract rational numbers efficiently using properties of operations.

Add and subtract rational numbers using a variety of strategies.

7.NS.A.1 7.NS.A.1.A 7.NS.A.1.B 7.NS.A.1.C 7.NS.A.1.D

Create a free account to access thousands of lesson plans.

Already have an account? Sign In

Topic B: Multiplying and Dividing Rational Numbers

Determine the rules for multiplying signed numbers.

7.NS.A.2.A 7.NS.A.2.C

Multiply signed rational numbers and interpret products in real-world contexts.

Determine the rules for dividing signed numbers.

7.NS.A.2.B 7.NS.A.2.C

Divide signed rational numbers and interpret quotients in real-world contexts.

Convert rational numbers to decimals using long division and equivalent fractions.

Multiply and divide with rational numbers using properties of operations.

7.NS.A.2 7.NS.A.2.C

Topic C: Using all Four Operations with Rational Numbers

Solve problems with rational numbers and all four operations.

Request a Demo

See all of the features of Fishtank in action and begin the conversation about adoption.

Learn more about Fishtank Learning School Adoption.

Contact Information

School information, what courses are you interested in, are you interested in onboarding professional learning for your teachers and instructional leaders, any other information you would like to provide about your school.

We Handle Materials So You Can Focus on Students

We've got you covered with rigorous, relevant, and adaptable math lesson plans for free

Operations on Rational Numbers

Operations on rational numbers are carried out in the same way as the arithmetic operations like addition, subtraction, multiplication, and division on integers and fractions. Arithmetic operations on rational numbers with the same denominators are easy to calculate but in the case of rational numbers with different denominators, we have to operate after making the denominators the same. Rational numbers are expressed in the form of fractions, but we do not call them fractions as fractions include only positive numbers, while rational numbers include both positive and negative numbers. Fractions are a part of rational numbers, while rational numbers are a broad category that includes other types of numbers.

In this lesson, we will explore operations on rational numbers by learning about addition, subtraction, multiplication, and division of rational numbers along with their properties.

What are Operations on Rational Numbers?

Operations on rational numbers refer to the mathematical operations carrying out on two or more rational numbers . A rational number is a number that is of the form p/q, where: p and q are integers, q ≠ 0. Some examples of rational numbers are: 1/2, −3/4, 0.3 (or) 3/10, −0.7 (or) −7/10, etc.

We know about fractions and how different operators can be used on different fractions. All the rules and principles that apply to fractions can also be applied to rational numbers. The one thing that we need to remember is that rational numbers also include negatives. So, while 1/5 is a rational number, it is true that −1/5 is also a rational number. There are four basic arithmetic operations with rational numbers: addition, subtraction, multiplication, and division. Let's learn about each in detail.

Addition of Rational Numbers

Adding rational numbers can be done in the same way as adding fractions. There are two cases related to the addition of rational numbers.

• Adding rational numbers with like denominators
• Adding rational numbers with different denominators

To add two or more rational numbers with like denominators, we simply add all the numerators and write the common denominator. For example, add 1/8 and 3/8. Let us understand this with the help of a number line.

• On the number line, we start from 1/8.
• We will take 3 jumps toward the right as we are adding 3/8 to it. As a result, we reach point 4/8. 1/8 + 3/8 = (1 + 3)/8 = 4/8 =1/2
• Thus, 1/8 + 3/8 = 1/2.

When rational numbers have different denominators, the first step is to make their denominators equivalent using the LCM of the denominators. Let's consider an example. Let us add the numbers −1/3 and 3/5

• Step 1: The denominators are different in the given numbers. Let's find the LCM of 3 and 5 to find the common denominator . LCM of 3 and 5 =15
• Step 2: Find the equivalent rational number with the common denominator. To do this, multiply −1/3 with 5 and 3/5 with 3 −1/3 × 5/5 and − 5/15 = 3/5 × 3/3 = 9/15.
• Step 3: Now the denominators are the same; simply add the numerators and then copy the common denominator. Always reduce your final answer to its lowest term. −1/3+3/5=(−1/3×5/5)+(3/5×3/3) =−5/5+9/15 =4/15

Subtraction of Rational Numbers

The process of subtraction of rational numbers is the same as that of addition. While subtracting two rational numbers on a number line, we move toward the left. Let us understand this method using an example. Subtract 1/2x−1/3x

• Step 1: Find the LCM of the denominators. LCM (2, 3) = 6.
• Step 2: Convert the numbers into their equivalents with 6 as the common denominator. 1/2x × 3/3 = 3/6x = 1/3x × 2/2 = 2/6x
• Step 3: Subtract the numbers you obtained in step 2.

Multiplication of Rational Numbers

Multiplication of rational numbers is similar to how we multiply fractions. To multiply any two rational numbers, we have to follow three simple steps. Let's multiply the following rational numbers: −2/3×(−4/5). The steps to find the solution are:

• Step 1: Multiply the numerators . (−2)×(−4)=8
• Step 2: Multiply the denominators. (3)×(5)=15
• Step 3: Reduce the resulting number to its lowest term. Since it's already in its lowest term, we can leave it as is. (−23)×(−45) = (−2)×(−4)/ (3)×(5) = 8/15

Division of Rational Numbers

We have learned in the whole number division that the dividend is divided by the divisor. Dividend÷Divisor=Dividend/Divisor. While dividing any two numbers, we have to see how many parts of the divisor are there in the dividend. This is the same for the division of rational numbers as well. Let us take an example to understand it in a better way. The steps to be followed to divide two rational numbers are given below:

• Step 1: Take the reciprocal of the divisor (the second rational number). 2x/9 = 9/2x
• Step 2: Multiply it to the dividend. −4x/3 × 9/2x
• Step 3: The product of these two numbers will be the solution. (−4x × 9) / (3 × 2x) = −6

Properties of Operations on Rational Numbers

Some of the properties that apply to the operations on rational numbers are listed below:

Related Articles

Check out a few more interesting articles related to the operations of rational numbers.

• Decimal Representation of Irrational Numbers
• Irrational Numbers
• Rationalize the Denominator
• Is pi a rational or Irrational Number

Important Notes

• Identity property does not hold true for subtraction and division of rational numbers.
• Closure property holds true for all four operations of rational numbers.
• Commutative property and associative property holds true for the addition and multiplication of rational numbers.
• Inverse property does not hold true for subtraction and division of rational numbers.x/y−(−x/y)≠0, x/y:y/x≠1

Examples of Operations of Rational Numbers

Example 1: Using the properties of rational numbers, determine the difference between −5/7 and 3/7.

The given rational numbers have a common denominator. Thus, we will subtract the numerators and retain the same denominator.

= −5/7−3/7 = (−5−3)/7 = −8/7

Therefore, the difference is −8/7.

Example 2: Saira uses 3/5 of the flour if she has to bake a full cake. How much flour will she use to bake 1/6 portion of the cake?

Total flour to bake a full cake = 3/5

Using operations on rational numbers,

Amount of flour used to bake1/6 portion of the cake = 3/5×1/6=(3×1)/(5×6)=3/30=1/10

Therefore, Saira would have to use 1/10 of the flour.

go to slide go to slide

Book a Free Trial Class

Practice Questions on Operations of Rational Numbers

Faqs on operations of rational numbers, what are the effects of different operations on rational and irrational numbers.

• The resultant of the addition of a rational number and an irrational number is an irrational number only as it doesn't affect the non-recurring and non-terminating nature of the irrationals.
• The sum of two rational numbers is a rational number.
• The sum of a rational number and an irrational number is irrational.
• The sum of two irrational numbers is an irrational number.
• The product of two rational numbers is a rational number.
• The product of a rational number and an irrational number is an irrational number.
• The product of two irrational numbers is an irrational number.

How Do You Perform Operations on Rational Numbers?

We perform the operations on rational numbers as follows:

• Addition and subtraction of rational numbers: In case, the denominators are the same, just add or subtract directly. In case, the denominators are different, take LCM to make the denominators the same and then solve.
• Multiplication of rational numbers: Multiply the numerators and multiply the denominators. Reduce the fraction so obtained in its lowest form.
• Division of Rational Numbers: Multiply the reciprocal of the divisor with the dividend.

What Are the Properties of Addition of Rational Numbers?

Properties of addition of rational numbers are described below:

• Addition of two rational numbers is also a rational number. (closure property)
• Three rational numbers can be added in any order. (Associative property)
• Two rational numbers can be rearranged internally without affecting the addition of numbers. (Commutative property)
• 0 is the additive identity of any rational number.
• Additive inverse of a rational number in the form of p/q is −pq.

Does the Identity Property Hold True for the Subtraction of Rational Numbers?

Identity property partially holds true in the case of subtraction of rational numbers, as x/y − 0 = x/y, but 0 − x/y ≠ x/y.

What Is the Rule for Subtracting Rational Numbers?

To subtract any two rational numbers

• Step 1: Check if the denominators are the same.
• Step 2: Make the denominators the same by taking the LCM of the denominators.
• Step 3: Subtract the given numbers by subtracting their numerators, leaving the denominator the same.

What Is the Difference Between Operations on Fractions and Operations on Rational Numbers?

In operations on rational numbers, we need to use the rules of operations on integers as well as operations on fractions, because rational numbers include negative numbers also. For positive rational numbers, the process of applying operations is the same as that of fractions.

Does the Inverse Property Hold True for the Division of Rational Numbers?

No, the inverse property does not hold true for the division of rational numbers, that's why we call it multiplicative inverse and not division inverse. Because if we divide x/y by y/x, we won't get 1 as the answer. Let's check. x/y ÷ y/x = x/y × x/y = x 2 /y 2 ≠16.

How To Add Two Negative Rational Numbers?

Let us take an example to understand how to add two negative rational numbers. Add: −1/2+(−3/4)

• Whenever there is a positive sign outside the bracket, we consider the sign of individual terms inside the bracket. So here, we can write it as −1/2 − 3/4.
• Now take the LCM of the denominators to make these terms like. LCM (2,4)=4
• Solve the numerators and write the final answer. −2/4 − 3/4 = −5/4

This is how we add two negative rational numbers.

How To Subtract Two Negative Rational Numbers?

Let us take an example to understand how to subtract two negative rational numbers. Subtract −3/7−(−4/3)

• Whenever there is a negative sign outside the bracket, we change the sign of individual terms inside the bracket. So here, we write −4/3 as +4/3.
• Now take the LCM of the denominators to make these terms like. LCM(7,3)=21
• −12/21 + 28/21. Solve the numerators and write the final answer. −12/21 + 28/21 = 16/21

This is how we subtract two negative rational numbers.

A community blog focused on educational excellence and equity

About the topic

Explore classroom guidance, techniques, and activities to help you meet the needs of ALL students.

most recent articles

Shaking Up High School Math

Executive Functions and Literacy Skills in the Classroom

Connecting and Communicating With Families to Help Break Down Barriers to Learning

Discover new tools and materials to integrate into you instruction.

Culture, Community, and Collaboration

Vertical Progression of Math Strategies – Building Teacher Understanding

“Can I have this? Can I have that?”

Find instructions and recommendations on how to adapt your existing materials to better align to college- and career-ready standards.

To Teach the Truth

Helping Our Students See Themselves and the World Through the Books They Read in Our Classrooms

Textbooks: Who Needs Them?

Learn what it means for instructional materials and assessment to be aligned to college- and career-ready standards.

Let’s Not Make Power ELA/Literacy Standards and Talk About Why We Didn’t

What to Consider if You’re Adopting a New ELA/Literacy Curriculum

Not Your Mom’s Professional Development

Delve into new research and perspectives on instructional materials and practice.

Summer Reading Club 2023

Synergy between College and Career Readiness Standards-Aligned Instruction and Culturally Relevant Pedagogy

Children Should be Seen AND Heard

• Submissions Guidelines
• About the Blog

Problem Solving With Rational Numbers

Digging into 7th-grade standard 7.NS.A.3

Welcome back to the Unfinished Learning in Middle School Math series, in which math educators Chrissy Allison and Becca Varon illustrate how to make some of the trickiest standards in grades 6-8 accessible for all students. Over the course of six blog posts, we’ll provide concrete examples of how math educators can address unfinished learning within the context of grade-level lessons, which in the long term will help prevent an entrenched pattern of over-remediation and below-grade-level teaching. You can read our introductory post here . In this fourth post, we will explore ways to “bridge the gap” with the 7th-grade standard 7.NS.A.3. 

Let’s take a look at the standard itself. As you read, consider how it applies to your everyday life.

Did anyone else almost have a heart attack when thinking about teaching this standard to middle schoolers with significant unfinished learning? We know we did!

In fact, years ago, when we started digging deeper into the topic of unfinished learning, 7.NS.A.3 was the standard that popped into my head because it relies heavily on skills and understanding from previous grade levels. 

Let’s take a moment to study and understand this standard:

Here’s the truth: We’d love students to have a deep understanding of every standard, and it’s important to work toward that every day in our classrooms. That said, there are some standards that have bigger implications for future learning and are a greater necessity for our everyday lives— and this is the case for standard 7.NS.A.3. 

We absolutely need this standard for life. It’s non-negotiable whether you’re creating a budget, managing your finances, or cooking a meal for your family—not to mention starting a business or moving into higher study of math. Engaging in common, everyday experiences involving operations with integers, fractions, and decimals is essential for students as they move into adulthood. This comfort with the math that will enable students to thrive financially and personally is a gift that not all of us were given in school ourselves.

Take a look at these tasks from Illustrative Mathematics. You’ll see the breadth of skills encompassed as part of 7.NS.A.3:

• Sharing Prize Money

After analyzing and solving several problems aligned to this culminating standard, we’ll admit we felt a bit overwhelmed thinking about the prerequisites from K-6 that are embedded in the work of 7.NS.A.3. 

Is your heart beating faster yet? Wondering if you need to reteach the entirety of the K-6 standards for students to be successful with 7.NS.A.3?

The short answer is no . This is not the time or place to start back at square one teaching students to add, subtract, multiply, and divide whole numbers, fractions, and decimals. You don’t have enough instructional time, nor is that the main goal of this standard. (Whew!) 

Okay, so if fluency with the operations isn’t the focus, then what is?

Let’s take a look at a problem from this 7th-grade lesson in the Illustrative Mathematics curriculum to shed some light.

When considering what it will take for students to engage in problem-solving and answering the questions correctly, three things come to mind:

1. Understanding the meaning of the problem. Familiarity with some of the vocabulary, such as “utility,” “kilowatt-hour,” “solar panel,” “credit,” and “charge,” will help students comprehend this real-life situation.

2. Planning a solution pathway. To answer each question, students must determine which calculations to perform, and with which numbers. They’ll need to recognize that subtraction makes sense for part 1, while division is called for in parts 2 and 3.

3. Calculating with positive and negative decimals. Finally, students will need to find the difference and quotients to answer this three-part question. 

While all three of these parts are necessary to be successful with this task, it’s important to remember that the aspect of rigor for 7.NS.A.3 is application. That means that the top priority is problem-solving, which means providing students with the opportunity to make sense of problems and persevere in solving them (Standard for Mathematical Practice 1) — not drill and kill with integers, fractions, and decimals.

So, let’s say that you open your curriculum and see this problem on the docket for next week, but you’ve diagnosed that your students have some unfinished learning when it comes to understanding and performing operations. 

Perhaps you’ve noticed students struggling to calculate fractions and decimals by hand in previous lessons, or maybe you have evidence from a formative or diagnostic assessment that students often apply an incorrect operation when problem-solving. Conceptual gaps have greater implications than skill-based gaps, so make sure you gauge students’ understanding, not just level of procedural fluency.

It’s natural to look at a rigorous problem and worry, “My kids can’t do that,” especially if you’ve put similar problems in front of students and it hasn’t gone well!

Over time, that negative reinforcement can lead teachers to a variety of responses that decrease students’ access to cognitively demanding, grade-level work. Those responses can include:

• Removing the problem from the lesson or not teaching it altogether
• Over-scaffolding the problem by walking students through it, step-by-step
• Adapting the problem to make it easier, perhaps by replacing fractions and decimals with whole numbers, without a plan to reach the standard’s full breadth later

What can you do to take action in a way that meets students where they are while keeping the rigor bar high and engaging students in deep thinking about math? Here are a few recommendations:

1. Bucket the critical prerequisites into two categories: skills, and concepts .

• Skills: identifying opposites, finding absolute value, operations with positive and negative integers, performing operations with whole numbers, fractions, decimals
• Concepts: opposites, integers, addition, subtraction, multiplication, division

2. To support students with the skills they need in the short term, allow use of a calculator.

• The focus of this standard is supporting students to be resourceful problem solvers , not human calculators. Spending days or weeks teaching “basic skills” is not a good use of your limited instructional time when it comes to teaching 7.NS.A.3. 
• This statement is backed by the National Council of Teachers of Mathematics (NCTM)’s Principles to Actions report (2014) , which lists as an unproductive belief:  “Calculators and other tools are at best a frill or distraction and at worst a crutch that keeps students from learning mathematics. Students should use these tools only after they have learned how to do procedures with paper and pencil.” Instead, a productive belief is that technology is a powerful tool that can support conceptual understanding, mathematical reasoning, and problem-solving.

3. Build understanding of concepts and vocabulary through visual representations and class discussion.

• Take advantage of “teachable moments” as they come up in grade-level lessons . For example, with the Buying & Selling Power problem, you could show a short video clip to build background knowledge about solar energy. To reinforce the relationship between multiplication and division, you could draw a picture to represent the charge of $0.12 per kilowatt hour, and have students discuss why it makes sense to divide $82.04 by $0.12 to answer question 2.
• Incorporate Math Talks in your daily or weekly lessons as a way to build understanding of concepts and continue working toward fluency . Fawn Nguyen’s free Math Talks site includes a list of resources, tips from her experiences implementing math tasks in her own classroom, and links to Math Talks you can use with your own students.

Who is ready to think?  

In conclusion, we must allow all students to be problem-solvers and have access to cognitively demanding tasks, even if they have unfinished learning with rational number operations. Though it might be counterintuitive, fluency with skills is not a prerequisite for having the opportunity to solve problems. The truth is that all students are ready to think.

• Mathematics
• Standards for Mathematical Practice

Leave a Reply Cancel reply

Your email address will not be published. Required fields are marked *

About the Author: Chrissy Allison is the founder and CEO of Mindful Math Coach whose core purpose is to support secondary math educators in providing positive and equitable learning experience for students of color. A former middle school math teacher, Chrissy became an instructional leader where she led the school's math team to increase summative assessment scores by 30% within three years. Her 5+ years experience serving as Director of Math Professional Learning & Content Design at educational nonprofits gives Chrissy unique insight and expertise about what it takes to shift teacher practice and move the needle with student learning. As host of The Mindful Math Podcast, Chrissy interviews experienced educators and shares her own advice and lessons learned to help teachers reach every learner while finding balance in their own lives. Chrissy lives in Chicago with her husband, Dan, and two children, Liviana and Otto. Learn more about Chrissy, access the podcast, and download free resources at www.mindfulmathcoach.com.

About the Author: Becca Varon is the Director of Math Content Learning at ANet. Prior to joining ANet, Becca taught 6th grade math and science in Glendale, Arizona and 5th grade math in Boston, Massachusetts. Becca now lives in Oakland, CA, where she continues to tutor local students in math.

Contextualizing Properties of Operations

Solving Multi-Step Equations

Stay in touch.

Like what you’re reading? Sign up to receive emails about new posts, free resources, and advice from educators.

Chapter 4, Lesson 3: Dividing Rational Numbers

• Extra Examples
• Self-Check Quizzes
• Data Updates
• Parent Student Study Guide

The resource you requested requires you to enter a username and password below:

Please read our Terms of Use and Privacy Policy before you explore our Web site. To report a technical problem with this Web site, please contact the site producer .

Chapter 3, Lesson 4: Dividing Rational Numbers

• Extra Examples
• Personal Tutor
• Self-Check Quizzes

The resource you requested requires you to enter a username and password below:

Please read our Terms of Use and Privacy Notice before you explore our Web site. To report a technical problem with this Web site, please contact the site producer .

• Inspiration

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons

Margin Size

• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

3.4: Rational Numbers

• Last updated
• Save as PDF
• Page ID 156271

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

Learning Objectives

After completing this section, you should be able to:

• Define and identify numbers that are rational.
• Simplify rational numbers and express in lowest terms.
• Add and subtract rational numbers.
• Convert between improper fractions and mixed numbers.
• Convert rational numbers between decimal and fraction form.
• Multiply and divide rational numbers.
• Apply the order of operations to rational numbers to simplify expressions.
• Apply density property of rational numbers.
• Solve problems involving rational numbers.
• Use fractions to convert between units.
• Define and apply percent.
• Solve problems using percent.

We are often presented with percentages or fractions to explain how much of a population has a certain feature. For example, the 6-year graduation rate of college students at public institutions is 57.6%, or 72/125. That fraction may be unsettling. But without the context, the percentage is hard to judge. So how does that compare to private institutions? There, the 6-year graduation rate is 65.4%, or 327/500. Comparing the percentages is straightforward, but the fractions are harder to interpret due to different denominators. For more context, historical data could be found. One study reported that the 6-year graduation rate in 1995 was 56.4%. Comparing that historical number to the recent 6-year graduation rate at public institutions of 57.6% shows that there hasn't been much change in that rate.

Definition: Rational Number

A rational number is any number that can be expressed as the ratio of two integers, where the denominator is not zero. In mathematical terms, a rational number is any number of the form \(\frac{a}{b}\), where \(a\) and \(b\) are integers and \(b \ne 0\). This includes integers (e.g., \(5\) can be written as \(\frac{5}{1}\), fractions (e.g., \(\frac{3}{4}\), and repeating or terminating decimals (e.g., 0.75 or 0.333...).

Forms of Rational Numbers

• Example: \(\frac{6}{11}\), \(-\frac{5}{7}\), \(\frac{2}{1}\)
• Example: 0.75 (which is \(\frac{3}{4}\))
• Example: 0.333... (which is \(\frac{1}{3}\))
• Example: 5 (which can be written as \(\frac{5}{1}\), -2 (which can be written as -\(\frac{2}{1}\))
• Example: \(2 \frac{1}{4}\) (which is \(\frac{9}{4}\))
• Example: 7:2 (which represents \(\frac{7}{2}\))
• Example: 50% (which is \(\frac{50}{100}\) or in reduced form \(\frac{1}{2}\))
• Example: { a/b | a, b ∈ ℤ, b ≠ 0 }

Summary of Forms

Defining and Identifying Numbers That Are Rational

A rational number (called rational since it is a ratio) is just a fraction where the numerator is an integer and the denominator is a non-zero integer. As simple as that is, they can be represented in many ways. It should be noted here that any integer is a rational number. An integer, n n , written as a fraction of two integers is \(\frac{n}{1}\).

In its most basic representation, a rational number is an integer divided by a non-zero integer, such as \(\frac{3}{12}\) (Figure \(\PageIndex{2}\)). Similarly, if in a group of 20 people, 5 are wearing hats, then \(\frac{5}{20}\) (Figure \(\PageIndex{3}\) ).

Another representation of rational numbers is as a mixed number, such as \(2 \frac{5}{8}\) ( Figure \(\PageIndex{4}\) ). This represents a whole number (2 in this case), plus a fraction (the 5 8 5 8 ).

Rational numbers may also be expressed in decimal form; for instance, as 1.34. When 1.34 is written, the decimal part, 0.34, represents the fraction 34 100 34 100 , and the number 1.34 is equal to 1 34 100 1 34 100 . However, not all decimal representations are rational numbers.

A number written in decimal form where there is a last decimal digit (after a given decimal digit, all following decimal digits are 0) is a terminating decimal , as in 1.34 above. Alternately, any decimal numeral that, after a finite number of decimal digits, has digits equal to 0 for all digits following the last non-zero digit.

All numbers that can be expressed as a terminating decimal are rational. This comes from what the decimal represents. The decimal part is the fraction of the decimal part divided by the appropriate power of 10. That power of 10 is the number of decimal digits present, as for 0.34, with two decimal digits, being equal to 34 100 34 100 .

Another form that is a rational number is a decimal that repeats a pattern, such as 67.1313… When a rational number is expressed in decimal form and the decimal is a repeated pattern, we use special notation to designate the part that repeats. For example, if we have the repeating decimal 4.3636…, we write this as 4. 36 ¯ 4. 36 ¯ . The bar over the 36 indicates that the 36 repeats forever.

Video \(\PageIndex{1}\)

Introduction to Fractions

If the decimal representation of a number does not terminate or form a repeating decimal, that number is not a rational number.

Square Roots

One class of numbers that is not rational is the square roots of integers or rational numbers that are not perfect squares , such as 10 10 and \(\sqrt{\frac{25}{6}}\) is the square root of the number \(a\) if \(a = b^{2}\). The notation for this if \(b = \sqrt{a}\), where the symbol, \(sqrt{}\), is the square root sign. An integer perfect square is any integer that can be written as the square of another integer. A rational perfect square is any rational number that can be written as a fraction of two integers that are perfect squares.

Sometimes you may be able to identify a perfect square from memory. Another process that may be used is to factor the number into the product of an integer with itself. Or a calculator (such as Desmos) may be used to find the square root of the number. If the calculator yields an integer, the original number was a perfect square.

Tech Check \(\PageIndex{1}\)

Using desmos to find the square root of a number.

When Desmos is used, there is a tab at the bottom of the screen that opens the keyboard for Desmos. The keyboard is shown below. On the keyboard (Figure \(\PageIndex{5}\) ) is the square root symbol ( ) ( ) . To find the square root of a number, click the square root key, and then type the number. Desmos will automatically display the value of the square root as you enter the number.

Example \(\PageIndex{1}\)

Identifying perfect squares.

Which of the following are perfect squares?

• We could attempt to find the perfect square by factoring. Writing all the factor pairs of 45 results in 1 × 45 , 3 × 15 1 × 45 , 3 × 15 , and 5 × 9 5 × 9 . None of the pairs is a square, so 45 is not a perfect square. Using a calculator to find the square root of 45, we obtain 6.708 (rounded to three decimal places). Since this was not an integer, the original number was not a perfect square.
• We could attempt to find the perfect square by factoring. Writing all the factor pairs of 144 results in 1 × 144 , 2 × 72 , 3 × 48 , 6 × 24 , 8 × 18 1 × 144 , 2 × 72 , 3 × 48 , 6 × 24 , 8 × 18 , and 12 × 12 12 × 12 . Since the last pair is an integer multiplied by itself, 144 is a perfect square. Alternately, using Desmos to find the square root of 144, we obtain 12. Since the square root of 144 is an integer, 144 is a perfect square.

Your Turn \(\PageIndex{1}\)

Determine if the following are perfect squares:

Example \(\PageIndex{2}\)

Identifying rational numbers.

Determine which of the following are rational numbers:

• 4.556 4.556
• 3 1 5 3 1 5
• 41 17 41 17
• 5 . 64 ¯ 5 . 64 ¯
• Since 73 is not a perfect square, its square root is not a rational number. This can also be seen when a calculator is used. Entering 73 73 into a calculator results in 8.544003745317 (and then more decimal values after that). There is no repeated pattern, so this is not a rational number.
• Since 4.556 is a decimal that terminates, this is a rational number.
• 3 1 5 3 1 5 is a mixed number, so it is a rational number.
• 41 17 41 17 is an integer divided by an integer, so it is a rational number.
• 5.646464... 5.646464... is a decimal that repeats a pattern, so it is a rational number.

Your Turn \(\PageIndex{2}\)

• \(\sqrt{13}\)
• \(-13.\overline{21}\)
• \(\frac{-48}{-16}\)
• \(-4 \frac{18}{19}\)

Simplifying Rational Numbers and Expressing in Lowest Terms

A rational number is one way to express the division of two integers. As such, there may be multiple ways to express the same value with different rational numbers. For instance, 4 5 4 5 and 12 15 12 15 are the same value. If we enter them into a calculator, they both equal 0.8. Another way to understand this is to consider what it looks like in a figure when two fractions are equal.

In Figure \(\PageIndex{6}\) , we see that 3 5 3 5 of the rectangle and 9 15 9 15 of the rectangle are equal areas.

They are the same proportion of the area of the rectangle. The left rectangle has 5 pieces, three of which are shaded. The right rectangle has 15 pieces, 9 of which are shaded. Each of the pieces of the left rectangle was divided equally into three pieces. This was a multiplication. The numerator describing the left rectangle was 3 but it becomes 3 × 3 3 × 3 , or 9, as each piece was divided into three. Similarly, the denominator describing the left rectangle was 5, but became 5 × 3 5 × 3 , or 15, as each piece was divided into 3. The fractions 3 5 3 5 and 9 15 9 15 are equivalent because they represent the same portion (often loosely referred to as equal).

This understanding of equivalent fractions is very useful for conceptualization, but it isn’t practical, in general, for determining when two fractions are equivalent. Generally, to determine if the two fractions \(\frac{a}{b}\) and \(\frac{c}{d}\) are equivalent, we check to see that \(a \times d = b \times c\). If those two products are equal, then the fractions are equal also.

Example \(\PageIndex{3}\)

Determining if two fractions are equivalent.

Determine if 12 30 12 30 and 14 35 14 35 are equivalent fractions.

Applying the definition, \(a = 12, b = 30, c = 14\) and \(d = 35\). So, \(a \times d = 12 \times 35 = 420\). Also, \( b \times c = 30 \times 14 = 420\). Since these values are equal, the fractions are equivalent.

Your Turn \(\PageIndex{3}\)

Determine if 8 14 8 14 and 12 26 12 26 are equivalent fractions.

That a × d = b × c a × d = b × c indicates the fractions \(\frac{a}{b}\) and \(\frac{c}{d}\) are equivalent is due to some algebra. One property of natural numbers, integers, and rational numbers (also irrational numbers) is that for any three numbers a , b , a , b , and c c with c ≠ 0 c ≠ 0 , if a = b a = b , then a / c = b / c a / c = b / c . In other words, when two numbers are equal, then dividing both numbers by the same non-zero number, the two newly obtained numbers are also equal. We can apply that to a × d a × d and b × c b × c , to show that \(\frac{a}{b}\) and \(\frac{c}{d}\) are equivalent if a × d = b × c a × d = b × c .

If a × d = b × c a × d = b × c , and c ≠ 0 , d ≠ 0 c ≠ 0 , d ≠ 0 , we can divide both sides by \(c\) and obtain the following: \(\frac{a \times d}{c} = \frac{b \times c}{c}\). We can divide out the c c on the right-hand side of the equation, resulting in \(\frac{a \times d}{c} = b\). Similarly, we can divide both sides of the equation by d d and obtain the following: \(\frac{a \times d}{d} = \frac{b \times c}{d}\). We can divide out d d the on the left-hand side of the equation, resulting in \(a = \frac{b \times c}{d}\). So, the rational numbers \(\frac{a}{c}\) and \(\frac{b}{d}\) are equivalent when a × d = b × c a × d = b × c .

Video \(\PageIndex{2}\)

Equivalent Fractions

Recall that a common divisor or common factor of a set of integers is one that divides all the numbers of the set of numbers being considered. In a fraction, when the numerator and denominator have a common divisor, that common divisor can be divided out . This is often called canceling the common factors or, more colloquially, as canceling .

To show this, consider the fraction 36 63 36 63 . The numerator and denominator have the common factor 3. We can rewrite the fraction as 36 63 = 12 × 3 21 × 3 36 63 = 12 × 3 21 × 3 . The common divisor 3 is then divided out, or canceled, and we can write the fraction as 12 × 3 21 × 3 = 12 21 12 × 3 21 × 3 = 12 21 . The 3s have been crossed out to indicate they have been divided out. The process of dividing out two factors is also referred to as reducing the fraction .

If the numerator and denominator have no common positive divisors other than 1, then the rational number is in lowest terms .

The process of dividing out common divisors of the numerator and denominator of a fraction is called reducing the fraction .

One way to reduce a fraction to lowest terms is to determine the GCD of the numerator and denominator and divide out the GCD. Another way is to divide out common divisors until the numerator and denominator have no more common factors.

Example \(\PageIndex{4}\)

Reducing Fractions to Lowest Terms

Express the following rational numbers in lowest terms:

• 36 48 36 48
• 100 250 100 250
• 51 136 51 136

Step 1: We can then rewrite the numerator and denominator by factoring 12 from both.

36 48 = 12 × 3 12 × 4 36 48 = 12 × 3 12 × 4

Step 2: We can now divide out the 12s from the numerator and denominator.

36 48 = 12 × 3 12 × 4 = 3 4 36 48 = 12 × 3 12 × 4 = 3 4

So, when 36 48 36 48 is reduced to lowest terms, the result is 3 4 3 4 .

Alternately, you could identify a common factor, divide out that common factor, and repeat the process until the remaining fraction is in lowest terms.

Step 1: You may notice that 4 is a common factor of 36 and 48.

Step 2: Divide out the 4, as in 36 48 = 4 × 9 4 × 12 = 4 × 9 4 × 12 = 9 12 36 48 = 4 × 9 4 × 12 = 4 × 9 4 × 12 = 9 12 .

Step 3: Examining the 9 and 12, you identify 3 as a common factor and divide out the 3, as in 9 12 = 3 × 3 3 × 4 = 3 4 9 12 = 3 × 3 3 × 4 = 3 4 . The 3 and 4 have no common positive factors other than 1, so it is in lowest terms.

Step 2: We can then rewrite the numerator and denominator by factoring 50 from both.

100 250 = 50 × 2 50 × 5 100 250 = 50 × 2 50 × 5 .

Step 3: We can now divide out the 50s from the numerator and denominator.

100 250 = 50 × 2 50 × 5 = 2 5 100 250 = 50 × 2 50 × 5 = 2 5

So, when 100 250 100 250 is reduced to lowest terms, the result is 2 5 2 5 .

Step 2: We can then rewrite the numerator and denominator by factoring 17 from both.

51 136 = 17 × 3 17 × 8 51 136 = 17 × 3 17 × 8

Step 3: We can now divide out the 17s from the numerator and denominator.

51 136 = 17 × 3 17 × 8 = 3 8 51 136 = 17 × 3 17 × 8 = 3 8

So, when 51 136 51 136 is reduced to lowest terms, the result is 3 8 3 8 .

Your Turn \(\PageIndex{4}\)

Express 252 840 252 840 and 17 51 17 51 in lowest terms.

Video \(\PageIndex{3}\)

Tech check \(\pageindex{2}\), using desmos to find lowest terms.

Desmos is a free online calculator . Desmos supports reducing fractions to lowest terms. When a fraction is entered, Desmos immediately calculates the decimal representation of the fraction. However, to the left of the fraction, there is a button that, when clicked, shows the fraction in reduced form.

Video \(\PageIndex{4}\)

Using Desmos to Reduce a Fraction

Adding and Subtracting Rational Numbers

Adding or subtracting rational numbers can be done with a calculator, which often returns a decimal representation, or by finding a common denominator for the rational numbers being added or subtracted.

Tech Check \(\PageIndex{3}\)

Using desmos to add rational numbers in fractional form.

To create a fraction in Desmos, enter the numerator, then use the division key (/) on your keyboard, and then enter the denominator. The fraction is then entered. Then click the right arrow key to exit the denominator of the fraction. Next, enter the arithmetic operation (+ or –). Then enter the next fraction. The answer is displayed dynamically (calculates as you enter). To change the Desmos result from decimal form to fractional form, use the fraction button (Figure 3.26) on the left of the line that contains the calculation:

Example \(\PageIndex{5}\)

Adding rational numbers using desmos.

Calculate 23 42 + 9 56 23 42 + 9 56 using Desmos.

Enter 23 42 + 9 56 23 42 + 9 56 in Desmos. The result is displayed as 0.70833333333 0.70833333333 (which is 0.708 3 ¯ 0.708 3 ¯ ). Clicking the fraction button to the left on the calculation line yields 17 24 17 24 .

Your Turn \(\PageIndex{5}\)

Calculate 124 297 124 297 + 3 125 3 125 in lowest terms.

Performing addition and subtraction without a calculator may be more involved. When the two rational numbers have a common denominator, then adding or subtracting the two numbers is straightforward. Add or subtract the numerators, and then place that value in the numerator and the common denominator in the denominator. Symbolically, we write this as \(\frac{a}{c} \pm \frac{b}{c} = \frac{a \pm b}{c}\) Figure \(\PageIndex{8}\), which shows \[ \frac{3}{20} + \frac{4}{20} = \frac{7}{20} \].

It is customary to then write the result in lowest terms.

If c c is a non-zero integer, then \(\frac{a}{c} \pm \frac{b}{c} = \frac{a \pm b}{c}\)

Example \(\PageIndex{6}\)

Adding rational numbers with the same denominator.

Calculate 13 28 + 7 28 13 28 + 7 28 .

Since the rational numbers have the same denominator, we perform the addition of the numerators, 13 + 7 13 + 7 , and then place the result in the numerator and the common denominator, 28, in the denominator. 13 28 + 7 28 = 13 + 7 28 = 20 28 13 28 + 7 28 = 13 + 7 28 = 20 28

Once we have that result, reduce to lowest terms, which gives 20 28 = 4 × 5 4 × 7 = 4 × 5 4 × 7 = 5 7 20 28 = 4 × 5 4 × 7 = 4 × 5 4 × 7 = 5 7 .

Your Turn \(\PageIndex{6}\)

Example \(\pageindex{7}\), subtracting rational numbers with the same denominator.

Calculate 45 136 − 17 136 45 136 − 17 136 .

Since the rational numbers have the same denominator, we perform the subtraction of the numerators, 45 − 17 45 − 17 , and then place the result in the numerator and the common denominator, 136, in the denominator. 45 136 − 17 136 − 45 − 17 136 = 28 136 45 136 − 17 136 − 45 − 17 136 = 28 136

Once we have that result, reduce to lowest terms, this gives 28 136 = 4 × 7 4 × 34 = 4 × 7 4 × 34 = 7 34 28 136 = 4 × 7 4 × 34 = 4 × 7 4 × 34 = 7 34 .

Your Turn \(\PageIndex{7}\)

When the rational numbers do not have common denominators, then we have to transform the rational numbers so that they do have common denominators. The common denominator that reduces work later in the problem is the LCM of the numerator and denominator. When adding or subtracting the rational numbers \(\frac{a}{b}\) and \(\frac{c}{d}\), we perform the following steps.

Step 1: Find LCM ( b , d ) LCM ( b , d ) .

Step 2: Calculate n = LCM ( b , d ) b n = LCM ( b , d ) b and m = L C M ( b , d ) d m = L C M ( b , d ) d .

Step 3: Multiply the numerator and denominator of \(\frac{a}{b}\) by n n , yielding a × n b × n a × n b × n .

Step 4: Multiply the numerator and denominator of \(\frac{c}{d}\) by m m , yielding c × m d × m c × m d × m .

Step 5: Add or subtract the rational numbers from Steps 3 and 4, since they now have the common denominators.

You should be aware that the common denominator is LCM ( b , d ) LCM ( b , d ) . For the first denominator, we have b × n = b × L C M ( b , d ) b = L C M ( b , d ) b × n = b × L C M ( b , d ) b = L C M ( b , d ) , since we multiply and divide LCM ( b , d ) LCM ( b , d ) by the same number. For the same reason, d × m = d × L C M ( b , d ) b = L C M ( b , d ) d × m = d × L C M ( b , d ) b = L C M ( b , d ) .

Example \(\PageIndex{8}\)

Adding rational numbers with unequal denominators.

Calculate 11 18 + 2 15 11 18 + 2 15 .

The denominators of the fractions are 18 and 15, so we label \(b = 18\) and \(d = 15\).

Step 1: Find LCM(18,15). This is 90.

Step 2 : Calculate n n and m m . n = 90 18 = 5 n = 90 18 = 5 and m = 90 15 = 6 m = 90 15 = 6 .

Step 3: Multiplying the numerator and denominator of 11 18 11 18 by n = 5 n = 5 yields 11 × 5 18 × 5 = 55 90 11 × 5 18 × 5 = 55 90 .

Step 4: Multiply the numerator and denominator of 2 15 2 15 by m = 6 m = 6 yields 2 × 6 15 × 6 = 12 90 2 × 6 15 × 6 = 12 90 .

Step 5: Now we add the values from Steps 3 and 4: 55 90 + 12 90 = 67 90 55 90 + 12 90 = 67 90 .

This is in lowest terms, so we have found that 11 18 + 2 15 = 67 90 11 18 + 2 15 = 67 90 .

Your Turn \(\PageIndex{8}\)

Calculate \(\frac{4}{9} + \frac{7}{12}\).

Example \(\PageIndex{9}\)

Subtracting rational numbers with unequal denominators.

Calculate 14 25 − 9 70 14 25 − 9 70 .

The denominators of the fractions are 25 and 70, so we label \(b = 25\) and \(d = 70\).

Step 1: Find LCM(25,70). This is 350.

Step 2: Calculate n n and m m : n = 350 25 = 14 and m = 350 70 = 5 m = 350 70 = 5 .

Step 3: Multiplying the numerator and denominator of 14 25 14 25 by n = 14 yields 14 × 14 25 × 14 = 196 350 14 × 14 25 × 14 = 196 350 .

Step 4: Multiplying the numerator and denominator of 9 70 9 70 by m = 5 m = 5 yields 9 × 5 70 × 5 = 45 350 9 × 5 70 × 5 = 45 350 .

Step 5: Now we subtract the value from Step 4 from the value in Step 3: 196 350 − 45 350 = 151 350 196 350 − 45 350 = 151 350 .

This is in lowest terms, so we have found that 14 25 − 9 70 = 151 350 14 25 − 9 70 = 151 350 .

Your Turn \(\PageIndex{9}\)

Calculate \(\frac{10}{99} - \frac{17}{300}\).

Video \(\PageIndex{5}\)

Adding and Subtracting Fractions with Different Denominators

Converting Between Improper Fractions and Mixed Numbers

One way to visualize a fraction is as parts of a whole, as in 5 12 5 12 of a pizza. But when the numerator is larger than the denominator, as in 23 12 23 12 , then the idea of parts of a whole seems not to make sense. Such a fraction is an improper fraction. That kind of fraction could be written as an integer plus a fraction, which is a mixed number . The fraction 23 12 23 12 rewritten as a mixed number would be 1 11 12 1 11 12 . Arithmetically, 1 11 12 1 11 12 is equivalent to 1 + 11 12 1 + 11 12 , which is read as “one and 11 twelfths.”

Improper fractions can be rewritten as mixed numbers using division and remainders. To find the mixed number representation of an improper fraction, divide the numerator by the denominator. The quotient is the integer part, and the remainder becomes the numerator of the remaining fraction.

Example \(\PageIndex{10}\)

Rewriting an improper fraction as a mixed number.

Rewrite 48 13 48 13 as a mixed number.

When 48 is divided by 13, the result is 3 with a remainder of 9. So, we can rewrite 48 13 48 13 as 3 9 13 3 9 13 .

Your Turn \(\PageIndex{10}\)

Rewrite \(\frac{96}{26}\) as a mixed number.

Video \(\PageIndex{6}\)

Converting an Improper Fraction to a Mixed Number Using Desmos

Similarly, we can convert a mixed number into an improper fraction. To do so, first convert the whole number part to a fraction by writing the whole number as itself divided by 1, and then add the two fractions.

Alternately, we can multiply the whole number part and the denominator of the fractional part. Next, add that product to the numerator. Finally, express the number as that product divided by the denominator.

Example \(\PageIndex{11}\)

Rewriting a mixed number as an improper fraction.

Rewrite 5 4 9 5 4 9 as an improper fraction.

Step 1: Multiply the integer part, 5, by the denominator, 9, which gives 5 × 9 = 45 5 × 9 = 45 .

Step 2: Add that product to the numerator, which gives 45 + 4 = 49 45 + 4 = 49 .

Step 3: Write the number as the sum, 49, divided by the denominator, 9, which gives 49 9 49 9 .

Your Turn \(\PageIndex{11}\)

Rewrite \(9 \frac{11}{12}\) as an improper fraction.

Tech Check \(\PageIndex{4}\)

Using desmos to rewrite a mixed number as an improper fraction.

Desmos can be used to convert from a mixed number to an improper fraction. To do so, we use the idea that a mixed number, such as 5 6 11 5 6 11 , is another way to represent 5 + 6 11 5 + 6 11 . If 5 + 6 11 5 + 6 11 is entered in Desmos, the result is the decimal form of the number. However, clicking the fraction button to the left will convert the decimal to an improper fraction, 61 11 61 11 . As an added bonus, Desmos will automatically reduce the fraction to lowest terms.

Converting Rational Numbers Between Decimal and Fraction Forms

Understanding what decimals represent is needed before addressing conversions between the fractional form of a number and its decimal form , or writing a number in decimal notation . The decimal number 4.557 is equal to 4 557 1,000 4 557 1,000 . The decimal portion, .557, is 557 divided by 1,000. To write any decimal portion of a number expressed as a terminating decimal, divide the decimal number by 10 raised to the power equal to the number of decimal digits. Since there were three decimal digits in 4.557, we divided 557 by 10 3 = 1000 10 3 = 1000 .

Decimal representations may be very long. It is convenient to round off the decimal form of the number to a certain number of decimal digits. To round off the decimal form of a number to n n (decimal) digits, examine the ( n + 1 n + 1 )st decimal digit. If that digit is 0, 1, 2, 3, or 4, the number is rounded off by writing the number to the n n th decimal digit and no further. If the ( n + 1 n + 1 )st decimal digit is 5, 6, 7, 8, or 9, the number is rounded off by writing the number to the n n th digit, then replacing the n n th digit by one more than the n n th digit.

Example \(\PageIndex{12}\)

Rounding off a number in decimal form to three digits.

Round 5.67849 to three decimal digits.

The third decimal digit is 8. The digit following the 8 is 4. When the digit is 4, we write the number only to the third digit. So, 5.67849 rounded off to three decimal places is 5.678.

Your Turn \(\PageIndex{12}\)

Example \(\pageindex{13}\), rounding off a number in decimal form to four digits.

Round 45.11475 to four decimal digits.

The fourth decimal digit is 7. The digit following the 7 is 5. When the digit is 5, we write the number only to the fourth decimal digit, 45.1147. We then replace the fourth decimal digit by one more than the fourth digit, which yields 45.1148. So, 45.11475 rounded off to four decimal places is 45.1148.

Your Turn \(\PageIndex{13}\)

To convert a rational number in fraction form to decimal form, use your calculator to perform the division.

Example \(\PageIndex{14}\)

Converting a rational number in fraction form into decimal form.

Convert 47 25 47 25 into decimal form.

Using a calculator to divide 47 by 25, the result is 1.88.

Your Turn \(\PageIndex{14}\)

Converting a terminating decimal to the fractional form may be done in the following way:

Step 1: Count the number of digits in the decimal part of the number, labeled n n .

Step 2: Raise 10 to the n n th power.

Step 3: Rewrite the number without the decimal.

Step 4: The fractional form is the number from Step 3 divided by the result from Step 2.

This process works due to what decimals represent and how we work with mixed numbers. For example, we could convert the number 7.4536 to fractional from. The decimal part of the number, the .4536 part of 7.4536, has four digits. By the definition of decimal notation, the decimal portion represents 4,536 10 4 = 4,536 10,000 4,536 10 4 = 4,536 10,000 . The decimal number 7.4536 is equal to the improper fraction 7 4,536 10,000 7 4,536 10,000 . Adding those to fractions yields 74,536 10,000 74,536 10,000 .

Example \(\PageIndex{15}\)

Converting from decimal form to fraction form with terminating decimals.

Convert 3.2117 to fraction form.

Step 1: There are four digits after the decimal point, so n = 4 n = 4 .

Step 2: Raise 10 to the fourth power, 10 4 = 10,000 10 4 = 10,000 .

Step 3: When we remove the decimal point, we have 32,117.

Step 4: The fraction has as its numerator the result from Step 3 and as its denominator the result of Step 2, which is the fraction 32,117 10,000 32,117 10,000 .

Your Turn \(\PageIndex{15}\)

The process is different when converting from the decimal form of a rational number into fraction form when the decimal form is a repeating decimal. This process is not covered in this text.

Multiplying and Dividing Rational Numbers

Multiplying rational numbers is less complicated than adding or subtracting rational numbers, as there is no need to find common denominators. To multiply rational numbers, multiply the numerators, then multiply the denominators, and write the numerator product divided by the denominator product. Symbolically, a b × c d = a × c b × d a b × c d = a × c b × d . As always, rational numbers should be reduced to lowest terms.

If b b and d d are non-zero integers, then a b × c d = a × c b × d a b × c d = a × c b × d .

Example \(\PageIndex{16}\)

Multiplying rational numbers.

Calculate 12 25 × 10 21 12 25 × 10 21 .

Multiply the numerators and place that in the numerator, and then multiply the denominators and place that in the denominator.

12 25 × 10 21 = 12 × 10 25 × 21 = 120 525 12 25 × 10 21 = 12 × 10 25 × 21 = 120 525

This is not in lowest terms, so this needs to be reduced. The GCD of 120 and 525 is 15.

120 525 = 15 × 8 15 × 35 = 8 35 120 525 = 15 × 8 15 × 35 = 8 35

Your Turn \(\PageIndex{16}\)

Video \(\pageindex{7}\).

Multiplying Fractions

As with multiplication, division of rational numbers can be done using a calculator.

Example \(\PageIndex{17}\)

Dividing decimals with a calculator.

Calculate 3.45 ÷ 2.341 using a calculator. Round to three decimal places if necessary.

Using a calculator, we obtain 1.473729175565997. Rounding to three decimal places we have 1.474.

Your Turn \(\PageIndex{17}\)

Before discussing the division of fractions without a calculator, we should look at the reciprocal of a number. The reciprocal of a number is 1 divided by the number. For a fraction, the reciprocal is the fraction formed by switching the numerator and denominator. For the fraction \(\frac{a}{b}\), the reciprocal is b a b a . An important feature of a number and its reciprocal is that its product is 1.

When dividing two fractions by hand, find the reciprocal of the divisor (the number that is being divided into the other number). Next, replace the divisor by its reciprocal and change the division into multiplication. Then, perform the multiplication. Symbolicallly, \(\frac{a}{b} \div \frac{c}{d} = \frac{a}{b} \times \frac{d}{c} = \frac{a \times d}{b \times c}\). As before, reduce to lowest terms.

If b , c b , c and d d are non-zero integers, then \(\frac{a}{b} \div \frac{c}{d} = \frac{a}{b} \times \frac{d}{c} = \frac{a \times d}{b \times c}\).

Example \(\PageIndex{18}\)

Dividing rational numbers.

• Calculate 4 21 ÷ 6 35 4 21 ÷ 6 35 .
• Calculate 1 8 ÷ 5 28 1 8 ÷ 5 28 .

Step 2: Multiply the first fraction by that reciprocal.

4 21 ÷ 6 35 = 4 21 × 35 6 = 140 126 4 21 ÷ 6 35 = 4 21 × 35 6 = 140 126

The answer, 140 126 140 126 is not in lowest terms. The GCD of 140 and 126 is 14. Factoring and canceling gives 140 126 = 14 × 10 14 × 9 = 10 9 140 126 = 14 × 10 14 × 9 = 10 9 .

Step 2: Multiply the first fraction by that reciprocal: 1 8 ÷ 5 28 = 1 8 × 28 5 = 28 40 1 8 ÷ 5 28 = 1 8 × 28 5 = 28 40

The answer, 28 40 28 40 , is not in lowest reduced form. The GCD of 28 and 40 is 4. Factoring and canceling gives 28 40 = 4 × 7 4 × 10 = 7 10 28 40 = 4 × 7 4 × 10 = 7 10 .

Your Turn \(\PageIndex{18}\)

• Calculate \(\frac{46}{175} \div \frac{69}{285}\).
• Calculate \(\frac{3}{40} \div \frac{42}{55}\)

Video \(\PageIndex{8}\)

Dividing Fractions

Summary of the Operations for Rational Numbers

Applying the Order of Operations to Simplify Expressions

The order of operations for rational numbers is the same as for integers, as discussed in Order of Operations. The order of operations makes it easier for anyone to correctly calculate and represent. The order follows the well-known acronym PEMDAS:

The first step in calculating using the order of operations is to perform operations inside the parentheses. Moving down the list, next perform all exponent operations moving from left to right. Next (left to right once more), perform all multiplications and divisions. Finally, perform the additions and subtractions.

Example \(\PageIndex{19}\)

Applying the order of operations with rational numbers.

Correctly apply the rules for the order of operations to accurately compute ( 5 7 − 2 7 ) × 2 3 ( 5 7 − 2 7 ) × 2 3 .

Step 1: To calculate this, perform all calculations within the parentheses before other operations.

( 5 7 − 2 7 ) × 2 3 = ( 3 7 ) × 2 3 ( 5 7 − 2 7 ) × 2 3 = ( 3 7 ) × 2 3

Step 2: Since all parentheses have been cleared, we move left to right, and compute all the exponents next.

( 3 7 ) × 2 3 = ( 3 7 ) × 8 ( 3 7 ) × 2 3 = ( 3 7 ) × 8

Step 3: Now, perform all multiplications and divisions, moving left to right.

( 3 7 ) × 8 = 24 7 ( 3 7 ) × 8 = 24 7

Your Turn \(\PageIndex{19}\)

Example \(\pageindex{20}\).

Correctly apply the rules for the order of operations to accurately compute 4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 2 3 + 5 ) ) 2 4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 2 3 + 5 ) ) 2 .

To calculate this, perform all calculations within the parentheses before other operations. Evaluate the innermost parentheses first. We can work separate parentheses expressions at the same time.

Step 1: The innermost parentheses contain 2 3 + 5 2 3 + 5 . Calculate that first, dividing after finding the common denominator.

4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 2 3 + 5 ) ) 2 = 4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 2 3 + 5 1 ) ) 2 = 4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 2 3 + 15 3 ) ) 2 = 4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 17 3 ) ) 2 4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 2 3 + 5 ) ) 2 = 4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 2 3 + 5 1 ) ) 2 = 4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 2 3 + 15 3 ) ) 2 = 4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 17 3 ) ) 2

Step 2: Calculate the exponent in the parentheses, ( 5 9 ) 2 ( 5 9 ) 2 .

4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 17 3 ) ) 2 = 4 + 2 3 ÷ ( ( 25 81 ) − ( 17 3 ) ) 2 4 + 2 3 ÷ ( ( 5 9 ) 2 − ( 17 3 ) ) 2 = 4 + 2 3 ÷ ( ( 25 81 ) − ( 17 3 ) ) 2

Step 3: Subtract inside the parentheses is done, using a common denominator.

4 + 2 3 ÷ ( ( 25 81 ) − ( 17 3 ) ) 2 4 + 2 3 ÷ ( ( 25 81 ) − ( 17 × 27 3 × 27 ) ) 2 4 + 2 3 ÷ ( ( 25 81 ) − ( 459 81 ) ) 2 4 + 2 3 ÷ ( ( − 434 81 ) ) 2 4 + 2 3 ÷ ( ( 25 81 ) − ( 17 3 ) ) 2 4 + 2 3 ÷ ( ( 25 81 ) − ( 17 × 27 3 × 27 ) ) 2 4 + 2 3 ÷ ( ( 25 81 ) − ( 459 81 ) ) 2 4 + 2 3 ÷ ( ( − 434 81 ) ) 2

Step 4: At this point, evaluate the exponent and divide.

4 + 2 3 ÷ ( ( − 434 81 ) ) 2 4 + 2 3 ÷ ( 188,356 6,561 ) = 4 + 2 3 × ( 6,561 188,356 ) = 4 + 2,187 94,178 4 + 2 3 ÷ ( ( − 434 81 ) ) 2 4 + 2 3 ÷ ( 188,356 6,561 ) = 4 + 2 3 × ( 6,561 188,356 ) = 4 + 2,187 94,178

Step 5: Add.

4 + 2,187 94,178 = 378,899 94,178 4 + 2,187 94,178 = 378,899 94,178

Had this been done on a calculator, the decimal form of the answer would be 4.0232 (rounded to four decimal places).

Your Turn \(\PageIndex{20}\)

Video \(\pageindex{9}\).

Order of Operations Using Fractions

Applying the Density Property of Rational Numbers

For any two distinct rational numbers \(a\) and \(b\) where \(a < b\), there exists a rational number \(c\) such that \(a < c < b\). This is called the density property of the rational numbers.

This means that no matter how close two rational numbers are, you can always find another rational number between them. In fact, there are infinitely many rational numbers that are possible between \(a\) and \(b\).

To find one of those numbers:

Step 1: Add the two rational numbers.

Step 2: Divide that result by 2.

The result is always a rational number. This follows what we know about rational numbers. If two fractions are added, then the result is a fraction. Also, when a fraction is divided by a fraction (and 2 is a fraction), then we get another fraction. This two-step process will give a rational number, provided the first two numbers are rational.

Example \(\PageIndex{21}\)

Demonstrate the density property of rational numbers by finding a rational number between 4 11 4 11 and 7 12 7 12 .

To find a rational number between 4 11 4 11 and 7 12 7 12 :

Step 1: Add the fractions.

4 11 + 7 12 = 4 × 12 11 × 12 + 7 × 11 12 × 11 = 48 132 + 77 132 = 125 132 4 11 + 7 12 = 4 × 12 11 × 12 + 7 × 11 12 × 11 = 48 132 + 77 132 = 125 132

Step 2: Divide the result by 2. Recall that to divide by 2, you multiply by the reciprocal of 2. The reciprocal of 2 is 1 2 1 2 , as seen below.

125 132 ÷ 2 = 125 132 × 1 2 = 125 264 125 132 ÷ 2 = 125 132 × 1 2 = 125 264

So, one rational number between 4 11 4 11 and 7 12 7 12 is 125 264 125 264 .

We could check that the number we found is between the other two by finding the decimal representation of the numbers. Using a calculator, the decimal representations of the rational numbers are 0.363636…, 0.473484848…, and 0.5833333…. Here it is clear that 125 264 125 264 is between 4 11 4 11 and 7 12 7 12 .

Solving Problems Involving Rational Numbers

Rational numbers are used in many situations, sometimes to express a portion of a whole, other times as an expression of a ratio between two quantities. For the sciences, converting between units is done using rational numbers, as when converting between gallons and cubic inches. In chemistry, mixing a solution with a given concentration of a chemical per unit volume can be solved with rational numbers. In demographics, rational numbers are used to describe the distribution of the population. In dietetics, rational numbers are used to express the appropriate amount of a given ingredient to include in a recipe. As discussed, the application of rational numbers crosses many disciplines.

Example \(\PageIndex{22}\)

Mixing soil for vegetables.

James is mixing soil for a raised garden, in which he plans to grow a variety of vegetables. For the soil to be suitable, he determines that 2 5 2 5 of the soil can be topsoil, but 2 5 2 5 needs to be peat moss and 1 5 1 5 has to be compost. To fill the raised garden bed with 60 cubic feet of soil, how much of each component does James need to use?

In this example, we know the proportion of each component to mix, and we know the total amount of the mix we need. In this kind of situation, we need to determine the appropriate amount of each component to include in the mixture. For each component of the mixture, multiply 60 cubic feet, which is the total volume of the mixture we want, by the fraction required of the component.

Step 1: The required fraction of topsoil is 2 5 2 5 , so James needs 60 × 2 5 60 × 2 5 cubic feet of topsoil. Performing the multiplication, James needs 60 × 2 5 = 120 5 = 24 60 × 2 5 = 120 5 = 24 (found by treating the fraction as division, and 120 divided by 5 is 24) cubic feet of topsoil.

Step 2: The required fraction of peat moss is also 2 5 2 5 , so he also needs 60 × 2 5 60 × 2 5 cubic feet, or 60 × 2 5 = 120 5 = 24 60 × 2 5 = 120 5 = 24 cubic feet of peat moss.

Step 3: The required fraction of compost is 1 5 1 5 . For the compost, he needs 60 × 1 5 = 60 5 = 12 60 × 1 5 = 60 5 = 12 cubic feet.

Your Turn \(\PageIndex{22}\)

Example \(\pageindex{23}\), determining the number of specialty pizzas.

At Bella’s Pizza, one-third of the pizzas that are ordered are one of their specialty varieties. If there are 273 pizzas ordered, how many were specialty pizzas?

One-third of the whole are specialty pizzas, so we need one-third of 273, which gives 1 3 × 273 = 273 3 = 91 1 3 × 273 = 273 3 = 91 , found by dividing 273 by 3. So, 91 of the pizzas that were ordered were specialty pizzas.

Your Turn \(\PageIndex{23}\)

Video \(\pageindex{10}\).

Finding a Fraction of a Total

Using Fractions to Convert Between Units

A common application of fractions is called unit conversion , or converting units , which is the process of changing from the units used in making a measurement to different units of measurement.

For instance, 1 inch is (approximately) equal to 2.54 cm. To convert between units, the two equivalent values are made into a fraction. To convert from the first type of unit to the second type, the fraction has the second unit as the numerator, and the first unit as the denominator.

From the inches and centimeters example, to change from inches to centimeters, we use the fraction 2.54 cm 1 in 2.54 cm 1 in . If, on the other hand, we wanted to convert from centimeters to inches, we’d use the fraction 1 in 2.54 cm 1 in 2.54 cm . This fraction is multiplied by the number of units of the type you are converting from , which means the units of the denominator are the same as the units being multiplied.

Example \(\PageIndex{24}\)

Converting liters to gallons.

It is known that 1 liter (L) is 0.264172 gallons (gal). Use this to convert 14 liters into gallons.

We know that 1 liter = 0.264172 gal. Since we are converting from liters, when we create the fraction we use, make sure the liter part of the equivalence is in the denominator. So, to convert the 14 liters to gallons, we multiply 14 by 1 gal 0.264172 gal / 1 liter 1 gal 0.264172 gal / 1 liter . Notice the gallon part is in the numerator since we’re converting to gallons, and the liter part is in the denominator since we are converting from liters. Performing this and rounding to three decimal places, we find that 14 liters is 14 liter × 0.264172 gal 1 liter = 3.69841 gal 14 liter × 0.264172 gal 1 liter = 3.69841 gal .

Your Turn \(\PageIndex{24}\)

Example \(\pageindex{25}\), converting centimeters to inches.

It is known that 1 inch is 2.54 centimeters. Use this to convert 100 centimeters into inches.

We know that 1 inch = 2.54 cm. Since we are converting from centimeters, when we create the fraction we use, make sure the centimeter part of the equivalence is in the denominator, 1 in 2.54 cm 1 in 2.54 cm . To convert the 100 cm to inches, multiply 100 by 1 in 2.54 cm 1 in 2.54 cm . Notice the inch part is in the numerator since we’re converting to inches, and the centimeter part is in the denominator since we are converting from centimeters. Performing this and rounding to three decimal places, we obtain 100 cm × 1 in 2.54 cm = 39.370 in 100 cm × 1 in 2.54 cm = 39.370 in . This means 100 cm equals 39.370 in.

Your Turn \(\PageIndex{25}\)

Video \(\pageindex{11}\).

Converting Units

Defining and Applying Percent

A percent is a specific rational number and is literally per 100. n n percent, denoted n n %, is the fraction n 100 n 100 .

Example \(\PageIndex{26}\)

Rewriting a percentage as a fraction.

Rewrite the following as fractions:

• Using the definition and \(n = 31\), 31% in fraction form is 31 100 31 100 .
• Using the definition and \(n = 93\), 93% in fraction form is 93 100 93 100 .

Your Turn \(\PageIndex{26}\)

Example \(\pageindex{27}\), rewriting a percentage as a decimal.

Rewrite the following percentages in decimal form:

• Using the definition and \(n = 54\), 54% in fraction form is 54 100 54 100 . Dividing a number by 100 moves the decimal two places to the left; 54% in decimal form is then 0.54.
• Using the definition and \(n = 83\), 83% in fraction form is 83 100 83 100 . Dividing a number by 100 moves the decimal two places to the left; 83% in decimal form is then 0.83.

Your Turn \(\PageIndex{27}\)

You should notice that you can simply move the decimal two places to the left without using the fractional definition of percent.

Percent is used to indicate a fraction of a total. If we want to find 30% of 90, we would perform a multiplication, with 30% written in either decimal form or fractional form. The 90 is the total , 30 is the percentage , and 27 (which is 0.30 × 90 0.30 × 90 ) is the percentage of the total .

n % n % of x x items is \(\frac{n}{100} \times x\). The x x is referred to as the total , the n n is referred to as the percent or percentage , and the value obtained from \(\frac{n}{100} \times x\) is the part of the total and is also referred to as the percentage of the total .

Example \(\PageIndex{28}\)

Finding a percentage of a total.

• Determine 40% of 300.
• Determine 64% of 190.
• The total is 300, and the percentage is 40. Using the decimal form of 40% and multiplying we obtain 0.40 × 300 = 120 0.40 × 300 = 120 .
• The total is 190, and the percentage is 64. Using the decimal form of 64% and multiplying we obtain 0.64 × 190 = 121.6 0.64 × 190 = 121.6 .

Your Turn \(\PageIndex{28}\)

• Determine 25% of 1,200.
• Determine 53% of 1,588.

In the previous situation, we knew the total and we found the percentage of the total. It may be that we know the percentage of the total, and we know the percent, but we don't know the total. To find the total if we know the percentage the percentage of the total, use the following formula.

If we know that n n % of the total is x x , then the total is \(\frac{100 \times x}{n}\) .

Example \(\PageIndex{29}\)

Finding the total when the percentage and percentage of the total are known.

• What is the total if 28% of the total is 140?
• What is the total if 6% of the total is 91?
• 28 is the percentage, so n = 28 . 28% of the total is 140, so x = 140 . Using those we find that the total was 100 × 140 28 = 500 100 × 140 28 = 500 .
• 6 is the percentage, so n = 6 n = 6 . 6% of the total is 91, so x = 91 x = 91 . Using those we find that the total was 100 × 91 6 = 1,516.6 100 × 91 6 = 1,516.6 .

Your Turn \(\PageIndex{29}\)

• What is the total if 25% of the total is 30?
• What is the total if 45% of the total is 360?

The percentage can be found if the total and the percentage of the total is known. If you know the total, and the percentage of the total, first divide the part by the total. Move the decimal two places to the right and append the symbol %. The percentage may be found using the following formula.

The percentage, n n , of b b that is a a is a b × 100 % a b × 100 % .

Example \(\PageIndex{30}\)

Finding the percentage when the total and percentage of the total are known.

Find the percentage in the following:

• Total is 300, percentage of the total is 60.
• Total is 440, percentage of the total is 176.
• The total is 300; the percentage of the total is 60. Calculating yields 0.2. Moving the decimal two places to the right gives 20. Appending the percentage to this number results in 20%. So, 60 is 20% of 300.
• The total is 440; the percentage of the total is 176. Calculating yields 0.4. Moving the decimal two places to the right gives 40. Appending the percentage to this number results in 40%. So, 176 is 40% of 440.

Your Turn \(\PageIndex{30}\)

• Total is 1,000, percentage of the total is 70.
• Total is 500, percentage of the total is 425.

Solve Problems Using Percent

In the media, in research, and in casual conversation percentages are used frequently to express proportions. Understanding how to use percent is vital to consuming media and understanding numbers. Solving problems using percentages comes down to identifying which of the three components of a percentage you are given, the total, the percentage, or the percentage of the total. If you have two of those components, you can find the third using the methods outlined previously.

Example \(\PageIndex{31}\)

Percentage of students who are sleep deprived.

A study revealed that 70% of students suffer from sleep deprivation, defined to be sleeping less than 8 hours per night. If the survey had 400 participants, how many of those participants had less than 8 hours of sleep per night?

The percentage of interest is 70%. The total number of students is 400. With that, we can find how many were in the percentage of the total, or, how many were sleep deprived. Applying the formula from above, the number who were sleep deprived was 0.70 × 400 = 280 0.70 × 400 = 280 ; 280 students on the study were sleep deprived.

Your Turn \(\PageIndex{31}\)

Example \(\pageindex{32}\), amazon prime subscribers.

There are 126 million users who are U.S. Amazon Prime subscribers. If there are 328.2 million residents in the United States, what percentage of U.S. residents are Amazon Prime subscribers?

We are asked to find the percentage. To do so, we divide the percentage of the total, which is 126 million, by the total, which is 328.2 million. Performing this division and rounding to three decimal places yields 126 328.2 = 0.384 126 328.2 = 0.384 . The decimal is moved to the right by two places, and a % sign is appended to the end. Doing this shows us that 38.4% of U.S. residents are Amazon Prime subscribers.

Your Turn \(\PageIndex{32}\)

Example \(\pageindex{33}\).

Evander plays on the basketball team at their university and 73% of the athletes at their university receive some sort of scholarship for attending. If they know 219 of the student-athletes receive some sort of scholarship, how many student-athletes are at the university?

We need to find the total number of student-athletes at Evander’s university.

Step 1: Identify what we know. We know the percentage of students who receive some sort of scholarship, 73%. We also know the number of athletes that form the part of the whole, or 219 student-athletes.

Step 2: To find the total number of student-athletes, use \(\frac{100 \times x}{n}\), with \(x = 219\) and \(n = 73\). Calculating with those values yields 100 × 219 73 = 300 100 × 219 73 = 300 .

So, there are 300 total student-athletes at Evander’s university

Your Turn \(\PageIndex{33}\)

Check your understanding.

• \(\sqrt {13}\)
• \(\frac{4}{3}\)
• \(0.2\overline {13}\)
• Express \(\frac{8}{30}\) in lowest terms.
• Calculate \(\frac{3}{8} + \frac{5}{12}\) and express in lowest terms.
• Convert 0.34 into fraction form.
• Convert \(\frac{47}{12}\) into a mixed number.
• Calculate \(\frac{2}{9} \times \frac{21}{22}\) and express in lowest terms.
• Calculate \(\frac{2}{5} \div \frac{3}{10} + \frac{1}{6}\).
• Identify a rational number between \(\frac{7}{8}\) and \(\frac{20}{21}\).
• Lina decides to save \(\frac{1}{8}\) of her take-home pay every paycheck. Her most recent paycheck was for $882. How much will she save from that paycheck?
• Determine 38% of 600.
• A microchip factory has decided to increase its workforce by 10%. If it currently has 70 employees, how many new employees will the factory hire? For the following exercises, identify which of the following are rational numbers.<\li>
• \(\sqrt{144}\)
• \(\sqrt{131}\) For the following exercises, reduce the fraction to lowest terms
• \(\frac{8}{10}\)
• \(\frac{30}{105}\)
• \(\frac{36}{539}\)
• \(\frac{231}{490}\)
• \(\frac{750}{17875}\) For the following exercises, do the indicated conversion. If it is a repeating decimal, use the correct notation.
• Convert \(\frac{25}{6}\) to a mixed number.
• Convert \(\frac{240}{53}\) to a mixed number.
• Convert \(2\frac{3}{8}\) to an improper fraction.
• Convert \(15\frac{7}{30}\) to an improper fraction.
• Convert \(\frac{4}{9}\) to decimal form.
• Convert \(\frac{13}{20}\) to decimal form.
• Convert \(\frac{27}{625}\) to decimal form.
• Convert \(\frac{11}{14}\) to decimal form.
• Convert \(0.23\) to fraction form and reduce to lowest terms.
• Convert \(3.8874\) to fraction form and reduce to lowest terms. For the following exercises, perform the indicated operations. Reduce to lowest terms.
• \(\frac{3}{5} + \frac{3}{10}\)
• \(\frac{3}{14} + \frac{8}{21}\)
• \(\frac{13}{36} - \frac{14}{99}\)
• \(\frac{13}{24} - \frac{4}{117}\)
• \(\frac{3}{7} \times \frac{21}{48}\)
• \(\frac{48}{143} \times \frac{77}{120}\)
• \(\frac{14}{27} \div \frac{7}{12}\)
• \(\frac{44}{75} \div \frac{484}{285}\)
• \(\left( \frac{3}{5} + \frac{2}{7} \right) \times \frac{10}{21}\)
• \(\frac{3}{8} \times \left( \frac{13}{12} - \frac{35}{36} \right)\)
• \(\left( \frac{3}{7} + \frac{5}{16} \right)^2 - \frac{5}{12}\)
• \(\frac{3}{8} \times \left( \frac{4}{9} - \frac{1}{8} \right)^2\)
• \(\left( \frac{2}{5} \times \left( \frac{7}{8} - \frac{2}{3} \right) \right)^2 \div \left( \frac{4}{9} + \frac{5}{6} \right) + \frac{7}{12}\)
• \(\left( \frac{1}{5} \div \left( \frac{3}{10} + \frac{11}{15} \right) \right) \times \left( \frac{2}{21} + \frac{5}{9} \right) - \left( \frac{8}{15} \div \frac{4}{33} \right)^2\)
• Find a rational number between \(\frac{8}{17}\) and \(\frac{15}{28}\).
• Find a rational number between \(\frac{3}{50}\) and \(\frac{13}{98}\).
• Find two rational numbers between \(\frac{3}{10}\) and \(\frac{19}{45}\).
• Find three rational numbers between \(\frac{5}{12}\) and \(\frac{175}{308}\).
• Convert 24% to fraction form and reduce completely.
• Convert 95% to fraction form and reduce completely.
• Convert 0.23 to a percentage.
• Convert 1.22 to a percentage.
• Determine 30% of 250.
• Determine 75% of 600.
• If 25% of a group is 41 members, how many members total are in the group?
• If 80% of the total is 60, how much is in the total?
• 13 is what percent of 20?
• 80 is what percent of 320?
• Professor Donalson’s history of film class has 60 students. Of those students, \(\frac{2}{5}\), say their favorite movie genre is comedy. How many of the students in Professor Donalson’s class name comedy as their favorite movie genre?
• Naia’s dormitory floor has 80 residents. Of those, \(\frac{3}{8}\), play Fortnight for at least 15 hours per week. How many students on Naia’s floor play Fortnight at least 15 hours per week?
• In Tara’s town there are 24,000 people. Of those, \(\frac{13}{100}\),are food insecure. How many people in Tara’s town are food insecure?
• Roughly \(\frac{39}{50}\) of air is nitrogen. If an enclosure holds 2,000 liters of air, how many liters of nitrogen should be expected in the enclosure?
• To make the dressing for coleslaw, Maddie needs to mix it with \(\frac{3}{5}\) mayonnaise and \(\frac{2}{5}\) apple cider vinegar. If Maddie wants to have 8 cups of dressing, how many cups of mayonnaise and how many cups of apple cider vinegar does Maddie need?
• Studying and going to class?
• Not sleeping?
• Roughly 20.9% of air is oxygen. How much oxygen is there in 200 liters of air?
• 65% of college students graduate within 6 years of beginning college. A first-year cohort at a college contains 400 students. How many are expected to graduate within 6 years?
• A 20% discount is offered on a new laptop. How much is the discount if the new laptop originally cost $700?
• Leya helped at a neighborhood sale and was paid 5% of the proceeds. If Leya is paid $171.25, what were the total proceeds from the neighborhood sale?
• Unit Conversion. 1 kilogram (kg) is equal to 2.20462 pounds. Convert 13 kg to pounds. Round to three decimal places, if necessary.
• Unit Conversion. 1 kilogram (kg) is equal to 2.20462 pounds. Convert 200 pounds to kilograms. Round to three decimal places, if necessary.
• Unit Conversion. There are 12 inches in a foot, 3 feet in a yard, and 1,760 yards in a mile. Convert 10 miles to inches. To do so, first convert miles to yards. Next, convert the yards to feet. Last, convert the feet to inches.
• Unit Conversion. There are 1,000 meters (m) in a kilometer (km), and 100 centimeters (cm) in a meter. Convert 4 km to centimeters.
• Determine the markup in dollars.
• The markup is what percent of the original cost? Round the percentage to one decimal place.
• Calculate the markup on the item, in dollars.
• What is the price for which Wegmans sells the item? This is the price Wegmans paid, plus the markup.
• Suppose Wegmans then offers a 25% discount on the sale price of the item (found in part b). In dollars, how much is the discount?
• Determine the price of the item after the discount (this is the sales price of the item minus the discount). Round to two decimal places.
• Is the new price after the markup and discount equal to the price Wegmans paid for the item? Explain.
• The original price was $150. After the 50% discount, what is the price of the item?
• The coupon is applied to the discount price. The coupon is for 25%. Find 25% of the sale price (found in part a).
• Find the price after applying the coupon (this is the value from part a minus the value from part b).
• The total amount saved on the item is the original price after all the discounts. Determine the total amount saved by subtracting the final price paid (part c) from the original price of the item.
• Determine the effective discount percentage, which is the total amount saved divided by the original price of the item.
• Was the effective discount percentage equal to 75%, which would be the 50% plus the 25%? Explain.
• Convert \( 0.\overline{7} \) to fraction form.
• Convert \( 0.\overline{45} \) to fraction form.
• Convert \( 3.1\overline{5} \) to fraction form.
• Convert \( 2.71\overline{94} \) to fraction form.

Skip to Main Content

• My Assessments
• My Curriculum Maps
• Communities
• Workshop Evaluation

Share Suggestion

Adding and subtracting rational numbers to solve problems.

• Printer Friendly Version
• Grade Levels 7th Grade
• Related Academic Standards CC.2.2.7.B.3 Model and solve real-world and mathematical problems by using and connecting numerical, algebraic, and/or graphical representations. CC.2.1.7.E.1 Apply and extend previous understandings of operations with fractions to operations with rational numbers.
• Assessment Anchors M07.A-N.1 Apply and extend previous understandings of operations to add, subtract, multiply, and divide rational numbers. M07.B-E.2 Solve real-world and mathematical problems using numerical and algebraic expressions, equations, and inequalities.
• Eligible Content M07.A-N.1.1.1 Apply properties of operations to add and subtract rational numbers, including real-world contexts. M07.B-E.2.1.1 Apply properties of operations to calculate with numbers in any form; convert between forms as appropriate. Example: If a woman making $25 an hour gets a 10% raise, she will make an additional 1/10 of her salary an hour, or $2.50, for a new salary of $27.50 an hour (or 1.1 × $25 = $27.50).
• Competencies

Students will compute and solve problems using rational numbers. They will:

• add and subtract rational numbers.
• solve real-world problems by adding and subtracting rational numbers.

Essential Questions

• How is mathematics used to quantify, compare, represent, and model numbers? 
• How are relationships represented mathematically?
• How can expressions, equations and inequalities, be used to quantify, solve, and/or analyze mathematical situations?
• What makes a tool and/or strategy appropriate for a given task?
• How can recognizing repetition or regularity assist in solving problems more efficiently?
• Rational Number: A number expressible in the form a / b, where a and b are integers, and b ≠ 0.
• Repeating Decimal: The decimal form of a rational number in which the decimal digits repeat in an infinite pattern.

60–90 minutes

Prerequisite Skills

• Lesson 2 Exit Ticket ( M-7-5-2_Exit Ticket and KEY.docx )
• Lesson 2 Small-Group Practice worksheet ( M-7-5-2_Small Group Practice and KEY.docx )
• Lesson 2 Expansion Worksheet ( M-7-5-2_Expansion and KEY.docx )
• Lesson 2 Computations Worksheet ( M-7-5-2_Computations and KEY.docx )
• Lesson 2 Word-Problem Examples ( M-7-5-2_Word Problem Examples and KEY.docx )

Related Unit and Lesson Plans

• Computing and Problem Solving with Rational Numbers
• Adding and Subtracting Rational Numbers on a Number Line
• Multiplying and Dividing Rational Numbers to Solve Problems

Related Materials & Resources

The possible inclusion of commercial websites below is not an implied endorsement of their products, which are not free, and are not required for this lesson plan.

• http://ca.ixl.com/math/grade-7/add-and-subtract-rational-numbers

IXL’s Grade 7 Add and Subtract Rational Numbers will give students additional practice with addition and subtraction of rational numbers.

• http://ca.ixl.com/math/grade-8/add-and-subtract-rational-numbers-word-problems

IXL’s Grade 8 Add and Subtract Rational Numbers: Word Problems will give students additional practice with solving word problems that involve rational numbers.

Formative Assessment

• The modeling activity can be used to assess students’ prior knowledge and understanding regarding addition of rational numbers with unlike denominators.
• Activity 1 can be used to assess each student’s ability to create a word problem involving the addition and/or subtraction of rational numbers while also understanding the solution process.
• Use the exit ticket to quickly evaluate student mastery.

Suggested Instructional Supports

Instructional Procedures

As students come into class, have them evaluate the following expressions using a number line.

• 0.75 + 2.95      (3.7)

Walk around the classroom as students are working through the example problems. Briefly discuss the answers and make sure students are comfortable modeling addition and subtraction of rational numbers on a number line before moving on.

“In Lesson 1 of this unit, we learned how to model addition and subtraction of rational numbers on a number line. Today, we are going to focus on performing these computations without the use of a number line. We will then use these skills to solve some real-life problems.”

Computations: Adding and Subtracting Rational Numbers

Before presenting some real-world problems, give students the opportunity to practice adding and subtracting rational numbers without the help of a number line. If necessary, go over the following examples together as a class.

the other is not. Often, when computing with fractions, it is best to write all numbers in fraction form.”

common denominator. The lowest common denominator in this case would be 5.”

numerators as indicated. The denominator will stay as is.”

it is, but we may want to rewrite the fraction as a mixed number to get a better idea of the value.”

Example 2:      − 4.64 + 9.85

• −4.64 + 9.85     “Think about the number line. Based on the signs of each addend, do

you suspect our final answer here will be positive or negative?” (Positive, the absolute value of 9.85 is larger than the absolute value of −4.64.)

• Think:            9.85 – 4.64    
•                             

Distribute the Lesson 2 Computations Worksheet ( M-7-5-2_Computations and KEY.docx ). Instruct students to complete the worksheet individually. Walk around the room as students work to be sure they are on task and performing the computations accurately. Following the worksheet, provide time for students to discuss any problems they encountered, questions they have, or revelations they discovered. First, ask students to describe the computation process used to find each sum or difference. Then confirm their understanding by restating the correct process.

Problem Solving with Rational Numbers

Now it is time for students to apply their understanding of computation to solving real-world problems. Discuss the following examples together as a class.

• “The amount by which his savings have decreased is equal to the difference of 1018.20 and 920.45, written as 1018.20 – 920.45 or 97.75. Thus, Steven’s savings decreased by $97.75.”

Distribute Lesson 2 Word-Problem Examples ( M-7-5-2_Word Problem Examples and KEY.docx ). Have students discuss the solution process for each example problem in a manner similar to the process demonstrated above. Confirm the correct ideas students express. Then say: “Look through the problems you just received. Think of how the example word problems can be solved. Do you need to add or subtract the rational numbers? How will you go about doing this for fractions with unlike denominators, or for mixed numbers?”

Activity 1: Write-Pair-Share

Ask the whole class to think of some real-world contexts that involve the addition or subtraction of rational numbers. Students should make a list of at least five real-world contexts and provide one word problem. Ask students to share their ideas with a partner. Give students about 5 minutes to share contexts and word problems. During this time, each partner may ask questions of the other partner. Then, the whole class can reconvene. One member from each partner group will share the list of real-world contexts and word problems with the class. The teacher may wish to post the real-world contexts and word problems in a file on the class Web page or use them as a classroom display. These student examples would then serve as a reference tool.

Have students complete Lesson 2 Exit Ticket ( M-7-5-2_Exit Ticket and KEY.docx ) at the close of the lesson to evaluate students’ level of understanding.

Use the suggestions in the Routine section to review lesson concepts throughout the school year. Use the small-group suggestions for any students who might benefit from additional instruction. Use the Expansion section to challenge students who are ready to move beyond the requirements of the standard.

• Routine: Throughout the school year, encourage students to be on the lookout for real-world situations that involve the addition or subtraction of rational numbers. Students can present the problems to the teacher, who will facilitate class participation in solving the rational number problem.
• Small Groups: Students who need additional practice can be pulled into small groups to work on the Lesson 2 Small-Group Practice worksheet ( M-7-5-3_Small Group Practice and KEY.docx ). Students can work on the matching together or work individually and compare answers when done.
• Expansion: Students who are prepared for a greater challenge can be given the Lesson 2 Expansion Worksheet ( M-7-5-2_Expansion and KEY.docx ). The worksheet includes more difficult numeric expressions involving rational numbers.

Related Instructional Videos

Insert template, information.