geometry assignment 1.1 undefined terms

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons

Margin Size

• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

1.1: Geometry Terms

• Last updated
• Save as PDF
• Page ID 2117

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

Define and use terms, including points, lines, planes, space, and postulates.

Geometric Definitions

A point is an exact location in space . It describes a location, but has no size. Examples are shown below:

A line is infinitely many points that extend forever in both directions. Lines have direction and location and are always straight .

Figure \(\ PageIndex{2}\)

A plane is a flat surface that contains infinitely many intersecting lines that extend forever in all directions. Think of a plane as a huge sheet of paper with no thickness that goes on forever.

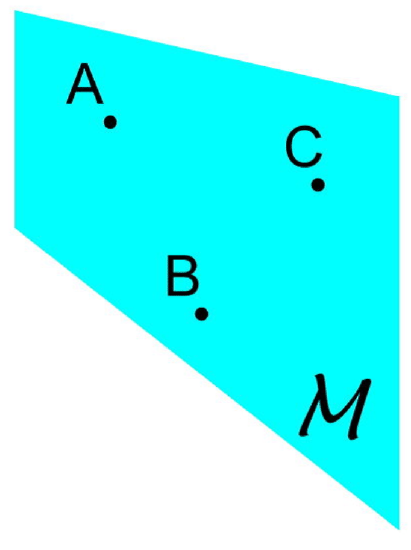
We can use point , line , and plane to define new terms.

Space is the set of all points extending in three dimensions . Think back to the plane. It extended in two dimensions, what we think of as up/down and left/right. If we add a third dimension, one that is perpendicular to the other two, we arrive at three-dimensional space.

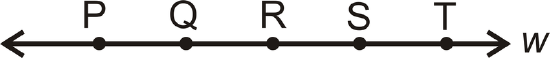
Points that lie on the same line are collinear . \(P\),\(Q\),\(R\),\(S\), and \(T\) are collinear because they are all on line \(w\). If a point U were located above or below line w, it would be non-collinear .

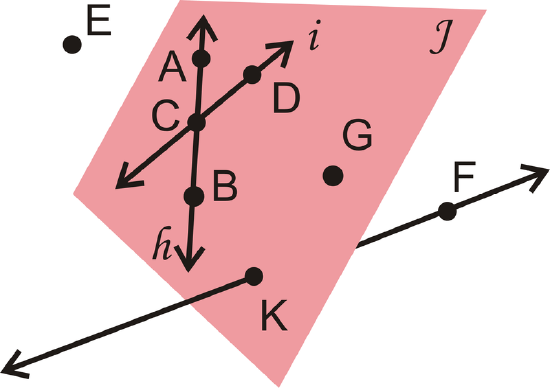
Points and/or lines within the same plane are coplanar . Lines h and i, and points \(A\),\(B\),\(C\),\(D\),\(G\), and \(K\) are coplanar in Plane \(J\). Line \(\overleftrightarrow{KF}\) and point \(E\) are non-coplanar with Plane \(J\).

An endpoint is a point at the end of a line segment . A line segment is a portion of a line with two endpoints. Or, it is a finite part of a line that stops at both ends. Line segments are labeled by their endpoints. Order does not matter.

A ray is a part of a line. It begins with an endpoint and extends forever away from the endpoint in one direction, perfectly straight. A ray is labeled by its endpoint and one other point on the ray. For rays, order does matter. When labeling, put the endpoint under the side WITHOUT the arrow.

An intersection is a point or set of points where lines, planes, segments, or rays overlap.

A postulate is a basic rule of geometry. Postulates are assumed to be true (rather than proven), much like definitions. The following is a list of some basic postulates.

Postulate #1: Given any two distinct points, there is exactly one (straight) line containing those two points.

Postulate #2: Given any three non-collinear points, there is exactly one plane containing those three points.

Postulate #3: If a line and a plane share two points, then the entire line lies within the plane.

Postulate #4: If two distinct lines intersect, the intersection will be one point.

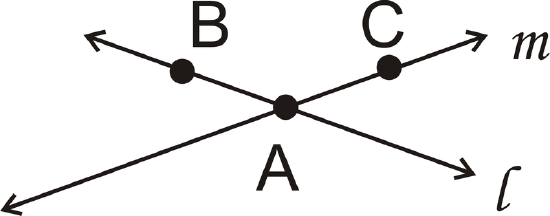
Lines \(I\) and \(m\) intersect at point \(A\).

Postulate #5: If two distinct planes intersect, the intersection will be a line.

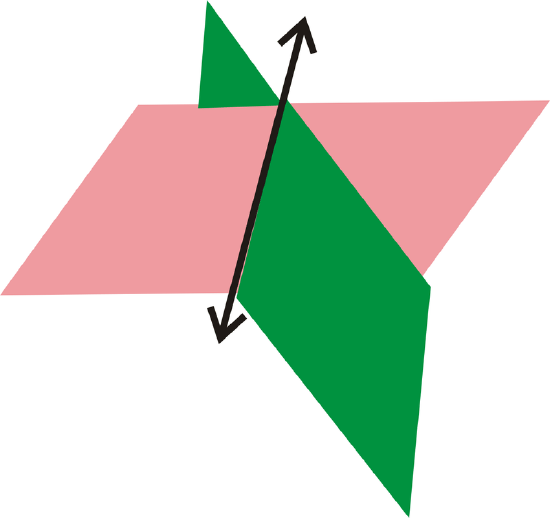
Figure \(\PageIndex{13}\)

When making geometric drawings, be sure to be clear and label all points and lines.

What if you were given a picture of a figure or object, like a map with cities and roads marked on it? How could you explain that picture geometrically?

Example \(\PageIndex{1}\)

What best describes San Diego, California on a globe: point, line, or plane?

A city is usually labeled with a dot, or point, on a globe.

Example \(\PageIndex{2}\)

Use the picture below to answer these questions.

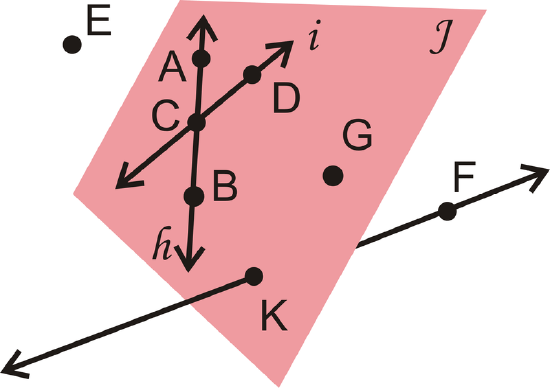
Figure \(\ PageIndex {14}\)

• List another way to label Plane \(J\).
• List another way to label line \(h\).
• Are \(K\) and \(F\) collinear?
• Are \(E\),\(B\) and \(F\) coplanar?
• Plane \(BDG\) is one possibility. Any combination of three coplanar points that are not collinear would be correct.
• \(\overleftrightarrow{AB}\). Any combination of two of the letters \(A\), \(B\), or \(C\) would also be correct.
• Yes, they both lie on \(\overleftrightarrow{KF}\).
• Yes, even though \(E\) is not in Plane \(J\), any three points make a distinct plane. Therefore, the three points create Plane \(EBF\).

Example \(\PageIndex{3}\)

What best describes a straight road that begins in one city and stops in a second city: ray, line, segment, or plane?

The straight road connects two cities, which are like endpoints. The best term is segment.

Example \(\PageIndex{4}\)

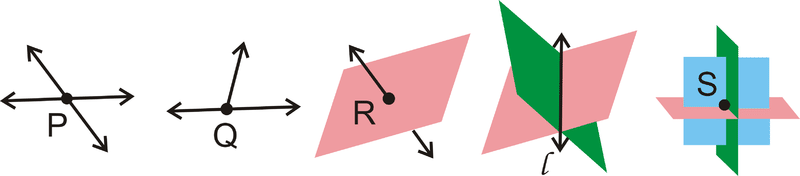
Answer the following questions about the picture.

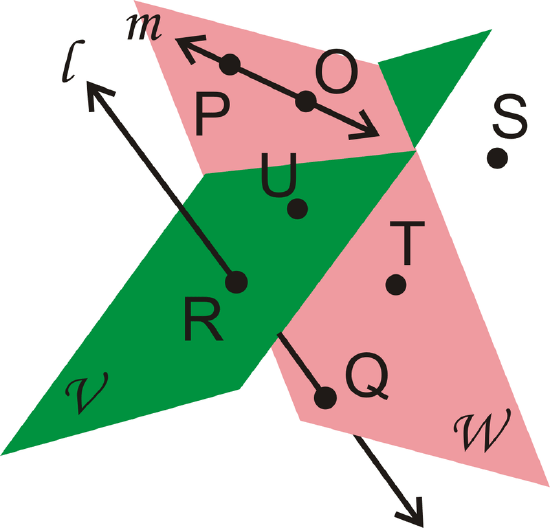
• Is line \(l\) coplanar with Plane \(V\), Plane \(W\), both, or neither?
• Are \(R\) and \(Q\) collinear?
• What point belongs to neither Plane V nor Plane \(W\)?
• List three points in Plane \(W\).
• Any combination of \(P\), \(O\), \(T\), and \(Q\) would work.

Example \(\PageIndex{5}\)

Draw and label a figure matching the following description: Line \(\overleftrightarrow{AB}\) and ray \(\overrightarrow{CD}\) intersect at point \(C)\. Then, redraw so that the figure looks different but is still true to the description.

Neither the position of A or B on the line, nor the direction that \(\overrightarrow{CD}\) points matter.

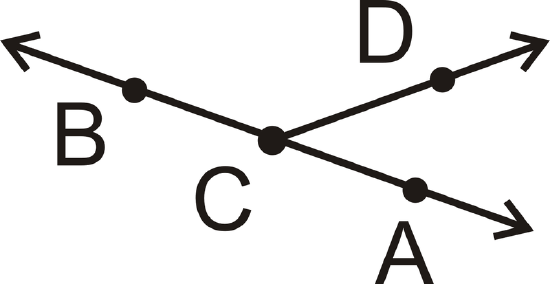
For the second part, this is one way to draw the diagram differently:

For questions 1-5, draw and label a figure to fit the descriptions.

• \(\overrightarrow{CD}\) intersecting \(\overline{AB}\) and Plane \(P\) containing \(\overline{AB}\) but not \(\overrightarrow{CD}\).
• Three collinear points \(A\), \(B\), and \(C\). \(B\) is also collinear with points \(D\) and \(E\).
• \(\overrightarrow{XY}\), \(\overrightarrow{XZ}\), and \(\overrightarrow{XW}\), such that \(\overrightarrow{XY}\) and \(\overrightarrow{XZ}\) are coplanar, but \(\overrightarrow{XW}\) is non-coplanar with both of the other rays.
• Two intersecting planes, \(P\) and \(Q\), with \(\overline{GH}\), where \(G\) is in plane \(P\) and \(H\) is in plane \(Q\).
• Four non-collinear points \(I\), \(J\), \(K\),and \(L\), with line segments connecting all points to each other.
• Name this line in five ways.

Figure \(\PageIndex{18}\)

• Name the geometric figure in three different ways.

Figure \(\PageIndex{19}\)

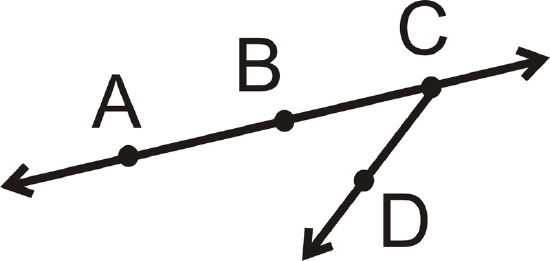
• Name the geometric figure in two different ways.

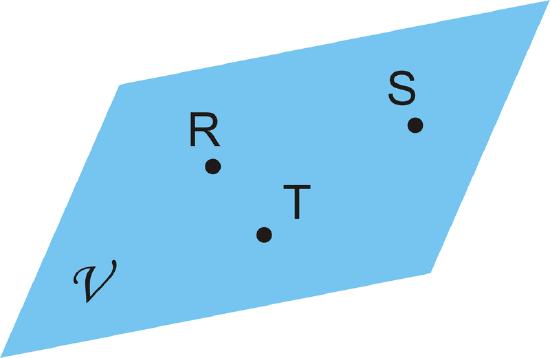
Figure \(\PageIndex{20}\)

• What is the best possible geometric model for a soccer field? Explain your answer.
• List two examples of where you see rays in real life.
• What type of geometric object is the intersection of a line and a plane? Draw your answer.
• What is the difference between a postulate and a theorem ?

For 13-16, use geometric notation to explain each picture in as much detail as possible.

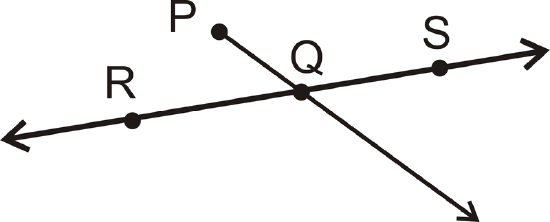
Figure \(\PageIndex{23}\)

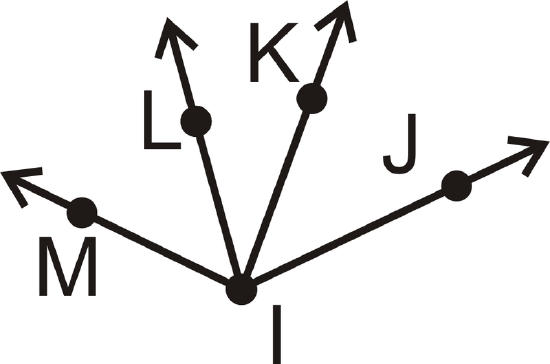
For 17-25, determine if the following statements are true or false.

• Any two points are collinear.
• Any three points determine a plane.
• A line is two rays with a common endpoint.
• A line segment is infinitely many points between two endpoints.
• A point takes up space.
• A line is one-dimensional.
• Any four points are coplanar.
• \(\overrightarrow{AB}\) could be read “ray \(AB\)” or “ray \(BA\).”
• \(\overleftrightarrow{AB}\) could be read “line \(AB\)” or “line \(BA\).”

Review (Answers)

To see the Review answers, open this PDF file and look for section 1.1.

Additional Resources

Interactive Element

Video: Basic Geometric Definitions Principles - Basic

Activities: Basic Geometric Definitions Discussion Questions

Study Aids: Basics of Geometry Study Guide

Practice: Geometry Terms

Real World: Basic Geometric Definitions

Undefined Terms in Geometry — Point, Line & Plane

Undefined terms definition

Four concepts in geometry can best be thought of as "described and not defined." In all branches of mathematics, some fundamental pieces cannot be defined, because they are used to define other, more complex pieces. In geometry, three  undefined terms  are the underpinnings of Euclidean geometry:

A fourth undefined term, set, is used in both geometry and set theory.

Even though these four terms are undefined, they can still be described. Mathematicians use descriptions of these four terms and work up from them, creating entire worlds of ideas like angles, polygons, Platonic solids, Cartesian graphs, and more.

Simply because these terms are formally undefined does not mean they are any less useful or valid than other terms that emerge from them. These four undefined terms are used extensively in theorems, proofs, and defining other words.

A  point  in geometry is described (but not defined) as a dimensionless location in space. A point has no width, depth, length, thickness -- no dimension at all. It is named with a capital letter: Point A ; Point B ; and so on.

Points in geometry are more like signal buoys on the vast, infinite ocean of geometric space than they are actual things. They tell you  where  a spot is, but are  not  the spot itself (even though we show them with a dot).

When you place two points on a plane, you can create a line.

A  line  is described (not defined) as the set of all collinear points between and extending beyond two given points. A line goes out infinitely past both points, but in geometry we symbolize this by drawing a short line segment, putting arrowheads on either end, and labeling two points on it.

The line is then identified by those two points. It can also be identified with a lowercase letter.

With only three points, you can create three different lines, and you can also describe a plane.

A  plane  is described as a flat surface with infinite length and width, but no thickness. It cannot be defined. A plane is formed by three points. For every three points in space, a unique plane exists.

A symbol of a plane in geometry is usually a trapezoid, to appear three-dimensional and understood to be infinitely wide and long. A single capital letter, or three points drawn on it, name the plane.

Modeling a plane in everyday life is tricky. Nothing will accurately substitute for a plane, because even the thinnest piece of paper, cookie sheet, or playing card still has some thickness. Also, all of these objects end abruptly at their edges. Planes do not end, and they have no thickness.

A  set  can be described as a collection of objects, in no particular order, that you are studying or mathematically manipulating. Sets can be all these things:

Physical objects like angles, rays, triangles, or circles

Numbers, like all positive even integers; proper fractions; or decimals smaller than 0.001

Other sets, like the set of all even numbers and the set of all multiples of five; the set of all acute angles and the set of all angles less than 15°

In geometry, we use sets to group numbers or items together to form a single unit, like all the triangles on a plane or all the straight angles on a coordinate grid. Sets are shown by using braces, { } , on either side of the set:

{0.1, 0.2, 0.3} for a set of three decimal numbers

{1, 2, 4, 8, 16…} for the infinite set of powers of two

{acute angles, obtuse angles, reflex angles, straight angles} for a set of angles found in plane geometry

{1, 2, 3…} for the infinite set of whole positive integers

{A, B, C…X, Y, Z} for the set of English alphabet letters

A set does not need to have a limit. The ellipsis (…) can indicate more terms between the start and end of the series, or it can indicate that the set between the braces continues on, infinitely.

A set does not need to ordered, like an array. You can write the first two sets shown above like this:

{0.2, 0.1, 0.3} or like this {0.3, 0.2, 0.1}

{4, 8, 16, 1, 2…} or like this {16, 2, 4, 1, 8…}

Undefined terms examples

Look on the floor of your bedroom. Mentally arrange a set of what you see. It might look like this:

{socks, gym shorts, left shoe, geometry textbook}

Look at a calendar. Mentally (or, better, jot down) a set of Saturday and Sunday dates. It might look like this:

{13, 14, 6, 20, 7, 27, 21, 28}

The order does not matter, but the set might be easier to work with in order from least to greatest:

{6, 7, 13, 14, 20, 21, 27, 28}

Lesson summary

Now that you have navigated your way through this lesson, you are able to identify and describe three undefined terms (point, line, and plane) that form the foundation of Euclidean geometry. You can also identify and describe the undefined term, set, used in geometry and set theory.

The Undefined Terms of Geometry

The undefined terms of geometry serve as the building blocks for defining other terms. There are three such undefined terms, including point, line, and plane. However, even if we don’t formally define them, we can, of course, describe these terms.

There are plenty of ways in which teachers can make teaching the undefined terms of geometry exciting, and today we bring you a few awesome tips to achieve this! Use these tips in your classroom and you’ll be sure to blend learning with fun!

Tips to Teach the Undefined Terms of Geometry

Undefined terms of geometry.

You can explain to students that the undefined terms of geometry are words or terms that don’t require any further explanation or elaborate description. Nonetheless, it’s good to provide a brief overview of them or a brief description to make sure that children understand them.

You may want to start by providing a brief description of each undefined term. Ask students to imagine a starry sky. Explain to students that a point is just like a star in the starry sky – it’s a dot indicating a location in space.

This dot can be tiny or large, but this doesn’t make any difference, as the point simply represents a location or position. So make sure to point out that a point has no size, length, width, or height. In the coordinate plane, we name points by ordered pairs (x, y).

Present a few examples of points by drawing a line on the whiteboard and placing a few points on it. Point out that we name the point with a capital letter. Then, ask the students to identify how many points there are on the line.

Ask students to think of that night sky full of stars again. Then, point out that a line is a straight path through the stars that extends in opposite directions with no end. So a line goes on forever in both directions and thus has infinite length and zero width and height.

Explain that a line doesn’t have endpoints and we draw it with arrowheads on both ends. The two-sided arrow is the symbol we use for lines. Lines are named by 2 points with a 2-sided arrow above the named points

Draw a line on the whiteboard with a few points on it and ask students to identify the names for the line.

Explain to students that a plane forms a flat surface that extends without end. A plane has two dimensions – length and width, and as it extends indefinitely, it has infinite length, width, and zero height, as well as infinite lines and points.

Point out that planes are named by an uppercase script letter (for example plane m ) or 3 non-collinear points on the plane. Add that non-collinear points on the plane are points that are not on the same line.

Explain that we draw a plane like a figure with four sides that looks like a parallelogram. Draw such a figure on the whiteboard to illustrate what a plane looks like. But make sure to point out that a plane has no boundaries, even though this diagram on the whiteboard has edges.

Line Segment

Describe a line segment as a part of a line between 2 endpoints. In other words, unlike lines, a line segment does not extend indefinitely in any direction and has a starting point and an endpoint.

Add that a line segment is named by its endpoints with a line without arrows above the names of the two endpoints. Draw a few line segments on the whiteboard and ask students to label and name them.

Finally, explain to students that a ray is a part of a line that consists of one endpoint and all the points on one side of the endpoint. A ray is named by its endpoint and another point on the ray.

Draw a few examples of rays on the whiteboard and ask students to identify and name them.

Additional Resources

If you have the technical means in your classroom, you can enrich your lesson with multimedia materials, such as videos. Videos will also come in handy for the visual representation of points, lines, and planes.

For instance, you can use this fun video that provides brief descriptions of the three undefined terms in geometry, followed by illustrative and colorful examples, such as dots on the tips of pencils or in the corners of boxes.

Activities to Practice the Undefined Terms of Geometry

Online activity.

This is a simple online activity by Khan Academy that will help students practice their skills at identifying and differentiating between the different undefined terms of geometry, as well as related terms. Introduce this activity at the end of your lesson.

Make sure there is a technical device for each student. Explain to students that they will do an online activity where they will have to answer several math challenges related to the undefined terms of geometry. They need to select the correct answer in the multiple-choice questions.

If a student gets stuck, they can also decide to use a hint or watch an explanatory video. In the end, provide some time for discussion and reflection. The activity is done individually, which makes it ideal for homeschooling parents as well.

This activity will allow students to reinforce their knowledge of points, lines, planes, as well as rays, line segments, and their skills at drawing these. To implement this activity in your classroom, the only thing you will need is to print out our free Assignment Worksheet .

Divide students into groups of 3 or 4 and hand out the worksheet. Make sure there is one copy per student. Explain to students that they need to work together with the members of their group to draw, name, and label the figures and terms in the assignment.

Provide around 15 minutes for groups to complete the worksheets. After this, open a class discussion and allow space for each group to share their work and the challenges they encountered while completing the tasks.

Before You Leave…

If you enjoyed these tips and resources, we have a whole lesson on teaching the undefined terms of geometry to 4th graders! So make sure to check out our worksheets and resources on teaching this. These are all pdf files, so you’ll be able to print them out easily:

• 1-2 Assignment 1 (PDF)
• 1-2 Assignment 2 (PDF)
• 1-2 Assignment SE (PDF)
• 1-2 Bell Work (PDF)
• 1-2 Bell Work SE (PDF)
• 1-2 Exit Quiz (PDF)
• 1-2 Exit Quiz SE (PDF)
• 1-2 Guide Notes SE (PDF)
• 1-2 Guide Notes TE (PDF)
• 1-2 Lesson Plan (PDF)
• 1-2 Online Activities (PDF)
• 1-2 Slide Show (PDF)

To get the Editable versions of these files, join us in the Math Teacher Coach community!

• 1-2 Assignment 1 ( Doc – Members Only )
• 1-2 Assignment 2 ( Doc – Members Only )
• 1-2 Bell Work ( Doc – Members Only )
• 1-2 Exit Quiz ( Doc – Members Only )
• 1-2 Guide Notes SE ( Doc – Members Only )
• 1-2 Guide Notes TE ( Doc – Members Only )
• 1-2 Lesson Plan ( Doc – Members Only )
• 1-2 Slide Show ( Doc – Members Only )
• 1-2 Online Activities ( Doc – Members Only )

This article is based on:

Unit 7 – Geometry

• 7-1 The Undefined Terms in Geometry
• 7-3 Parallel and Perpendicular Lines
• 7-4 Measuring and Sketching Angles
• 7-5 Addition of Angle Measures
• 7-6 Symmetry in 2D Figures
• 7-7 Triangles
• 7-8 Quadrilaterals

Do You Need More Math Resources?

If you’re looking for more materials to structure your classes and teach math to children of all ages, sign up for our emails and get loads of free lessons and content!

Feel free to also check out our blog – you’ll find plenty of awesome resources that you can use in your class! And if you’re ready to become a member, simply sign up at Math Teacher Coach !

Share this:

• Click to share on Twitter (Opens in new window)
• Click to share on Facebook (Opens in new window)

• school Campus Bookshelves
• menu_book Bookshelves
• perm_media Learning Objects
• login Login
• how_to_reg Request Instructor Account
• hub Instructor Commons

Margin Size

• Download Page (PDF)
• Download Full Book (PDF)
• Periodic Table
• Physics Constants
• Scientific Calculator
• Reference & Cite
• Tools expand_more
• Readability

selected template will load here

This action is not available.

1.2: Book 1 Definitions

• Last updated
• Save as PDF
• Page ID 105366

• Wayne Bishop
• California State University, Los Angeles

\( \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} \)

\( \newcommand{\id}{\mathrm{id}}\) \( \newcommand{\Span}{\mathrm{span}}\)

( \newcommand{\kernel}{\mathrm{null}\,}\) \( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\) \( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\) \( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\inner}[2]{\langle #1, #2 \rangle}\)

\( \newcommand{\Span}{\mathrm{span}}\)

\( \newcommand{\id}{\mathrm{id}}\)

\( \newcommand{\kernel}{\mathrm{null}\,}\)

\( \newcommand{\range}{\mathrm{range}\,}\)

\( \newcommand{\RealPart}{\mathrm{Re}}\)

\( \newcommand{\ImaginaryPart}{\mathrm{Im}}\)

\( \newcommand{\Argument}{\mathrm{Arg}}\)

\( \newcommand{\norm}[1]{\| #1 \|}\)

\( \newcommand{\Span}{\mathrm{span}}\) \( \newcommand{\AA}{\unicode[.8,0]{x212B}}\)

\( \newcommand{\vectorA}[1]{\vec{#1}}      % arrow\)

\( \newcommand{\vectorAt}[1]{\vec{\text{#1}}}      % arrow\)

\( \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } \)

\( \newcommand{\vectorC}[1]{\textbf{#1}} \)

\( \newcommand{\vectorD}[1]{\overrightarrow{#1}} \)

\( \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} \)

\( \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} \)

• A point is that which has no part. [Essentially meaningless as a definition. From a modern perspective, there must be some undefined terms. This is one.]
• A line is breadthless length. [Again, this is no definition. It is another undefined term.]
• The extremities of a line are points. [ Endpoints . Formally, something about "betweenness".]
• A straight line is a line which lies evenly with the points on itself. [Say what? Curves are "lines" here but what is "straight"? In Chapter 5, we will define it formally but it won’t seem natural!]
• A surface is that which has length and breadth only. [A region in the (plane) geometry.]
• The extremities of a surface are lines. [Edges of a polygon or polyhedron are line segments .]
• A plane surface is a surface which lies evenly with the straight lines on itself. [See the above.]
• A plane angle is the inclination to one another of two lines in a plane which meet one another and do not lie in a straight line. [Modern? An angle is the union of two rays with a common initial point.]
• And when the lines containing the angle are straight, the angle is called rectilineal . [As opposed to an angle determined by the intersection of two "smooth" curves; i.e. the intersection of their tangents at the point of intersection. We will need these in the "Poincaré disk model" for hyperbolic geometry.]
• When a straight line set up on a straight line makes the adjacent angles equal to one another, each of the equal angles is right , and the straight line standing on the other is called a perpendicular to that on which it stands. [Modern? Perpendicular lines are lines that intersect to form congruent, adjacent angles. The angles they form are called right angles.]
• An obtuse angle is an angle greater than a right angle. [A real definition.]
• An acute angle is an angle less than a right angle. [Two in a row!]
• A boundary is that which is an extremity of anything. [Your sarcasm here?]
• A figure is that which is contained by any boundary or boundaries. [I.e., closed and bounded.]
• A circle is a plane figure contained by one line [curve] such that all the straight lines falling upon it from one point among those lying within the figure are equal to one another. [Another, "Say what?"!]
• And the point is called the center of the circle. [Another real definition!]
• A diameter of the circle is any straight line drawn through the center and terminated in both directions by the circumference of the circle, and such a straight line also bisects the circle. [Omit "circumference”.]
• A semicircle is the figure contained by the diameter and the circumference cut off by it. And the center of the semicircle is the same as that of the circle. [As in #17, "circumference" implies the circle itself, the set of points equidistant from a given point; circumference actually means the linear measure of the circle.]
• Rectilineal figures are those which are contained by straight lines, trilateral figures being those contained by three, quadrilateral those contained by four, and multilateral those contained by more than four straight lines. [Real definitions but we’d say polygon and, in case of three, triangle , but trilateral is used as is quadrangle and \(n\) -gon if we wish to specify the number of sides, \(n .]\)
• Of trilateral figures, an equilateral triangle is that which has its three sides equal, an isosceles triangle that which has two of its sides alone equal, and a scalene triangle that which has its three sides unequal.
• Further, of trilateral figures, a right-angled triangle is that which has a right angle, an obtuse-angled triangle that which has an obtuse angle, and an acute angled triangle that which has its three angles acute. [For us, right triangle, obtuse triangle , and acute triangle .]
• Of quadrilateral figures, a square is that which is both equilateral and right-angled; an oblong that which is right-angled but not [necessarily] equilateral; a rhombus that which is equilateral but not [necessarily] right-angled; and a rhomboid that which has its opposite sides and angles equal to one another but is neither equilateral nor right-angled. And let quadrilaterals other than these be called trapezia . [Never used!]
• Parallel straight lines are straight lines which, being in the same plane and being produced indefinitely in both directions, do not meet one another in either direction. [Do they exist? Yes (but not in elliptic geometry), but what does "infinite" mean? Formally, it is the Archimedean Property: Given two segments, enough copies of the shorter one exceeds the longer one (independent of their lengths.]

Postulates. [ Axiom and postulate are interchangeable terms from the modern perspective, statements accepted as true without proof. Not in some universal sense, only in the context under consideration]

Let the following be postulated:

• To draw a straight line from any point to any point. [Hereafter, Axiom 1 : Two points determine...]
• To produce a finite straight line continuously in a straight line. [Lines are "infinite" - Subsumed under the Ruler Postulate ]
• To describe a circle with any center and distance. [Axiomatically, a point and a radius determine a unique circle and circles behave as they should. ’Nuff said.]
• That all right angles are equal to one another. [Subsumed under the Protractor Postulate .]
• That, if a straight line falling on two straight lines make the interior angles on the same side less than two right angles, the two straight lines, if produced indefinitely, meet on that side on which are the angles less than the two right angles. [ Euclid’s 5th Postulate , traditional form. Far more common is Playfair’s form: Given a line and a point not on the line, there is at most one line on the given point that is parallel to the given line. At most one? Maybe not even one?! No, but by proof, not by axiom].

Common Notions [From a modern perspective, these follow from set theory and properties of real numbers that can also be developed axiomatically. If we were to do so, we would never get done!]

• Things which are equal to the same thing are also equal to one another. [ Read : Congruence is transitive.]
• If equals be added to equals, the wholes are equal.
• If equals be subtracted from equals, the remainders are equal.
• Things which coincide with one another are equal to one another.
• The whole is greater than the part.

Proposition 1. On a given finite straight line to construct an equilateral triangle.

[Note the use of the infinitive as a command, "to construct". Think ancient Latin (or modern Spanish). Also "finite straight line" means line segment .]

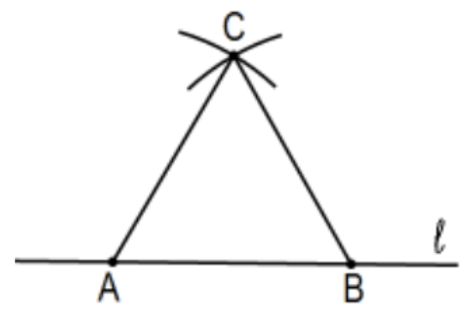
Construction : Choose any 2 points on the line, say A and B. Let C be the intersection of circles (A; AB) and (B; AB), the circles centered at \(A\) and \(B\) both of radius \(\mathrm{AB}\) (only one of the two intersections of these two circles to be more precise). The triangle determined by the 3 points, \(\triangle \mathrm{ABC}\) is equilateral.

Proof : By construction, all 3 sides are congruent, the definition of equilateral. [But how do we know the circles have to intersect? Can they "slip through" one another without intersecting? No, but there are geometries where they might. Without bothering to state them, we assume (as did the Greeks) that our axioms assure validity of such "continuity" considerations.]

Proposition 2 . To place at a given point (as an extremity) a straight line equal to a given straight line

As stated, this really means "to construct"; i.e., use a compass to copy a line segment at any point on another line. It’s immediate from the Ruler Postulate ; exactly one point (with direction) for any given length.

Proposition 3 . Given two unequal straight lines, to cut off from the greater a straight line equal to the less. [This is also part of the Ruler Postulate so nothing to prove, at least from a modern viewpoint.]

Proposition 4 . If two triangles have the two sides equal to two sides respectively, and have the angles contained by the equal straight lines equal, they will also have the base equal to the base, the triangle will be equal to the triangle, and the remaining angles will be equal to the remaining angles respectively, namely those which the equal sides subtend. [That is, Side-Angle-Side ( SAS ) that we will assume as our triangle congruence axiom. It is proved in Book 1 but "superposition", the underlying assumption of the ancient Greek proof, is tantalizingly obvious so don’t blame the Greeks too much for not realizing the need for an axiom; in our case, the SAS Axiom. ]

Proposition 5 . In isosceles triangles, the angles at the base are equal to one another, and, if the equal straight lines be produced further, the angles under the base will be equal to one another.

[See the Introduction and the introduction to this chapter and say "the base angles are congruent ."]

Proposition 6 . If in a triangle two angles be equal to one another, the sides which subtend the equal angles will also be equal to one another. [The converse of the "base angles of an isosceles triangle theorem"; as usually stated: In a triangle, sides opposite congruent angles are congruent.]

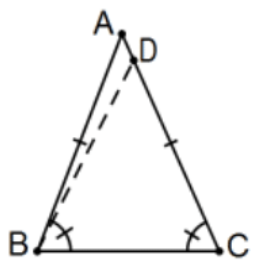
Given: \(\triangle \mathrm{ABC}\) with \(\angle \mathrm{B} \cong \angle \mathrm{C} \underline{\text { Prove: }} \mathrm{AB} \cong \mathrm{AC}\) .

Proof : Take D to be the point along ray CA with \(C D \cong B A\) (by the Ruler Postulate). Now \(\triangle \mathrm{DCB} \cong \triangle \mathrm{ABC}\) by SAS so \(\angle \mathrm{DBC} \cong \angle \mathrm{ACB}\) by cpctc and, by hypothesis and transitivity, \(\angle \mathrm{DBC} \cong \angle \mathrm{ABC}\) . By the Protractor Postulate, ray \(\mathrm{BD}\) is the same ray as BA so, by the Ruler Postulate again, \(\mathrm{D}=\mathrm{A}\) (exactly the same point!), and \(\mathrm{CA} \cong \mathrm{BA}\) just by renaming \(\mathrm{CD}\) as CA. QED.

Proposition 7 . Given two straight lines constructed on a straight line (from its extremities) and meeting in a point, there cannot be constructed on the same straight line (from its extremities), and on the same side of it, two other straight lines meeting in another point and equal to the former two respectively, namely each to that which has the same extremity with it. [This, together with Prop 8 , is usually known as the Side-Side-Side CongruenceTheorem ( SSS ). Formally, if two triangles have three pairs of sides congruent, the triangles themselves are congruent.]

Proposition 8 (SSS) . If two triangles have the two sides equal to two sides respectively, and have also the base equal to the base, they will also have the angles equal which are contained by the equal straight lines.

Given: \(\mathrm{AB} \cong \mathrm{EF}, \mathrm{AC} \cong \mathrm{EG}, \mathrm{BC} \cong \mathrm{FG}\)

Prove: \(\quad \triangle \mathrm{ABC} \cong \triangle \mathrm{EFG}\)

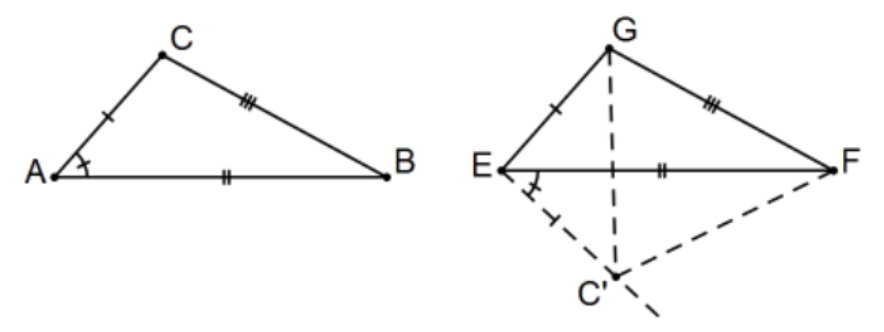
Proof : Copy \(\angle \mathrm{A}\) at E but on the opposite side of line EF than \(G\) (Protractor Postulate). Along that ray, let \(C^{\prime}\) be the point such that \(\mathrm{EC}^{\prime} \cong \mathrm{AC}\) so, by the given hypothesis and transitivity, \(\triangle \mathrm{EC}{ }^{\prime} \mathrm{G}\) is isosceles with base angles at \(C^{\prime}\) and \(G\) . Moreover, \(\triangle \mathrm{ABC} \cong \triangle \mathrm{EFC}^{\prime}\) by \(\mathrm{SAS}\) so \(\mathrm{FC}^{\prime} \cong \mathrm{BC}\) by cpctc so \(\triangle \mathrm{FGC}{ }^{\prime}\) is also isosceles, also with base angles at \(\mathrm{C}^{\prime}\) and \(\mathrm{G}\) . Adding congruent angles, we have \(\angle \mathrm{EC}^{\prime} \mathrm{F} \cong \angle \mathrm{EGF}\) . Thus \(\triangle \mathrm{EFC}{ }^{\prime} \cong \triangle \mathrm{EFG}\) by \(\mathrm{SAS}\) and \(\triangle \mathrm{ABC} \cong \triangle \mathrm{EFG}\) by transitivity. QED.

As long as it is done logically, there is nothing magic about following Euclid’s Elements Book 1 in its original sequence and it is convenient to look at Prop 23 at this point:

Proposition 23. On a given straight line and at a point on it to construct a rectilineal angle equal to a given rectilineal angle. [That is, copy an angle using straightedge and compass.]

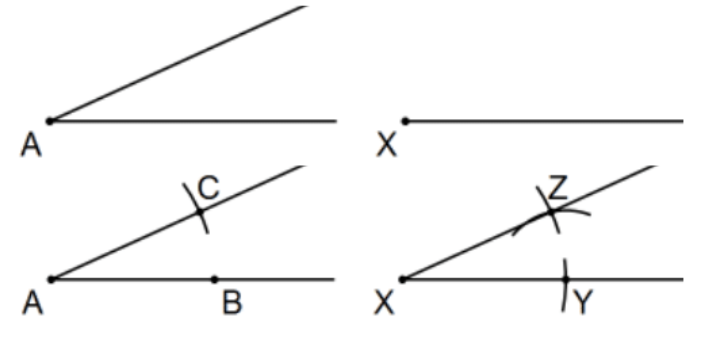
Construction : To copy \(\angle \mathrm{A}\) at point \(\mathrm{X}\) on the side given, choose any point \(B \neq A\) on one of the rays of \(\angle A\) an use that as the radius for circles centered at \(A\) and at \(\mathrm{X}\) to establish points \(\mathrm{C}\) and \(\mathrm{Y}\) and an arc in the vicinity of (not yet determined \(\mathrm{Z}\) ) Construct the circle with center at \(\mathrm{Y}\) of radius \(\mathrm{BC}\) to establish point \(\mathrm{Z}\) . Ray XZ is the desired angle at point \(\mathrm{X}\) .

Proof : \(\triangle \mathrm{ABC} \cong \triangle \mathrm{XYZ}\) by \(\mathrm{SSS}\) so \(\angle \mathrm{A} \cong \angle \mathrm{X}\) by cpctc. \(\mathrm{QED} .\)

Proposition 9 . To bisect a given rectilinear angle.

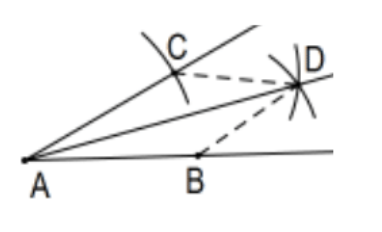
Construction : Choose a point, say B, on one of the rays of the angle and determine point \(C\) on the other ray by constructing the circle \((A ; A B)\) , the circle with center A of radius \(A B\) . With length \(r>(1 / 2) m(B C)\) , construct circles \((B ; r)\) and \((C ; r)\) and let \(D\) be their point of intersection (farthest from \(A\) , as pictured). Ray AD is the desired angle bisector. [Do NOT construct (or even indicate) segments BD and CD or any such dashed segments in these constructions; they are only indicated to clarify the proof.]

Proof : \(\triangle \mathrm{ABD} \cong \triangle \mathrm{ACD}\) by \(\mathrm{SSS}\) so that \(\angle \mathrm{BAD} \cong \angle \mathrm{CAD}\) by cpctc and, by definition of angle bisector, we’re done. [Why not until now? In fact, there is an \(\mathrm{SAS}\) proof for this so that it could have been done before SSS. Can you see it?]

Proposition 10 . To bisect a given finite straight line. [That is, construct the midpoint of a line segment .]

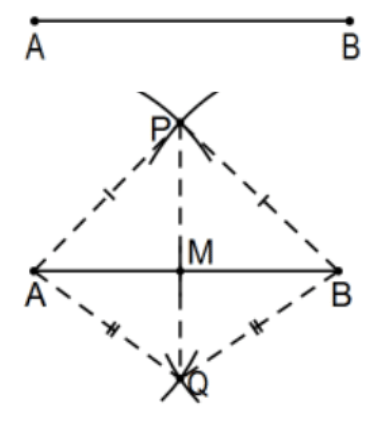
Construction : [Note: This does not say the perpendicular bisector, only the point that bisects; i.e., the midpoint. After the traditional construction, the perpendicular bisector of the segment to determine its midpoint, we will look at another that emphasizes this point. The problem is that some of you will say "perpendicular bisector" when you only mean one of "perpendicular" or "bisector". Words have meaning so use them correctly and meaningfully.]

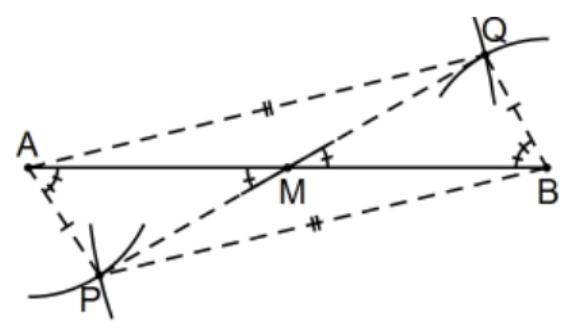
By means of circles, of the same radii, each greater than \((1 / 2) \mathrm{m}(A B)\) , construct two points, each equidistant from the endpoints; in this case, \(A\) and \(B\) are the endpoints and \(P\) and \(Q\) are the points, each equidistant from \(A\) and \(B\) . Finally, let \(M\) be the intersection of lines \(A B\) and PQ. [As in Prop 9, DON’T include the pictured dashed segments, only the construction . They are only there for clarity in the proof.]

Proof : By SSS, \(\triangle \mathrm{APQ} \cong \triangle \mathrm{BPQ}\) so that \(\angle \mathrm{APQ} \cong \angle \mathrm{BPQ}\) by cpctc. [Note: More clever would’ve been to say "From the proof of Prop 9 , we have \(\angle \mathrm{APQ} \cong \angle \mathrm{BPQ}\) . Can you see it?] In any case, we now have \(\triangle \mathrm{APM}\) \(\cong \triangle \mathrm{BPM}\) by \(\mathrm{SAS}\) so that \(\mathrm{AM} \cong \mathrm{BM}\) by cpctc and \(\mathrm{M}\) is the midpoint of \(\mathrm{AB}\) . QED.

Note: A useful extension of the proof above is:

Theorem : A point is on the perpendicular bisector of a line segment if and only if it is equidistant from each of its endpoints (PS 1, #20).]

[As promised, here is a construction you probably would not have thought of that we can’t prove yet but only because we don’t yet have AAS or congruence of vertical angles. If we did...

Construction : Consider two pairs of circles with centers A and B of the same radii large enough to intersect at points \(\mathrm{P}\) and Q. Let \(\mathrm{M}\) be the intersection of lines \(\mathrm{AB}\) and \(\mathrm{PQ}\) ; then \(\mathrm{M}\) is the desired point.

Proof : \(\triangle \mathrm{ABQ} \cong \triangle \mathrm{BAP}\) by SSS so \(\angle \mathrm{ABQ} \cong \angle \mathrm{BAP}\) by cpctc. But \(\angle \mathrm{ABQ}=\angle \mathrm{MBQ}\) (i.e., exactly the same angle) and \(\angle \mathrm{BAP}=\angle \mathrm{MAP}\) with \(\angle A M P \cong \angle B M Q\) because they are vertical angles. Now \(\triangle A M P \cong \triangle B M Q\) by \(A A S\) and, by cpctc, \(A M \cong B M\) as desired. QED.]

Proposition 11 . To draw a straight line at right angles to a given straight line from a given point on it. [Construct a perpendicular at a given point on a given line. Note: Do not use the other intersection of these two circles. Why not? Is PQ the only perpendicular to the line on the point P? Yes. Why?]

Proof : See PS 1, #9.

Proposition 12 . To a given infinite straight line, from a given point which is not on it, to draw a perpendicular straight line. [Construct a perpendicular to a line from a point not on the line. Is it the perpendicular to the line on the point? Not yet; not until after Prop 16.]

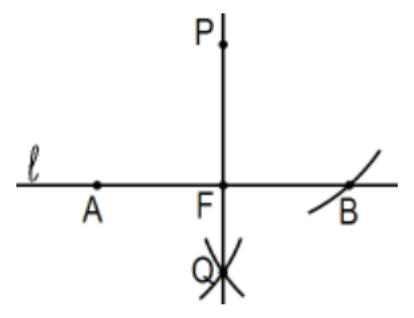
Proof : See PS 1, #8.

Proposition 13 . If a straight line [ray] set up on a straight line make angles, it will make either two right angles or angles equal to two right angles. [Nothing to prove; it is axiomatic by the Protractor Postulate.]

Proposition 14 . If with any straight line, and at a point on it, two straight lines not lying on the same side make the adjacent angles equal to two right angles, the two straight lines will be in a straight line with one another. [Here either. It is also a consequence of the Protractor Postulate.]

Proposition 15 . If two straight lines cut one another, they make the vertical angles equal to one another.

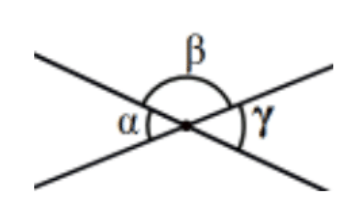
Proof : Viewed most simply: \(\alpha+\beta=180^{\circ}=\beta+\gamma\) implying \(\alpha=\gamma . \quad\) QED.

Proposition 16 . [ Exterior Angle Theorem (neutral geometry form). KNOW IT!] In any triangle, if one of the sides be produced, the exterior angle is greater than either of the nonadjacent interior angles.

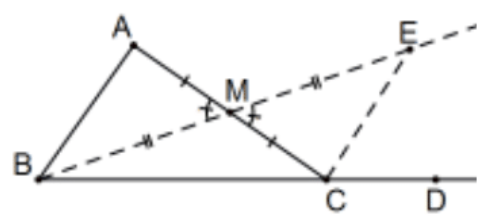
Proof : Let ray BM be the median on \(\mathrm{B}\) [ \(\mathrm{M}\) is the midpoint of segment AC] and let \(\mathrm{E}\) be along that ray such that \(\mathrm{EM} \cong \mathrm{BM}\) . Conclude that \(\angle \mathrm{A} \cong \angle \mathrm{ACE}<\angle \mathrm{ACD}\) . Why does this prove that \(\angle \mathrm{B}<\angle \mathrm{ACD}\) ? QED.

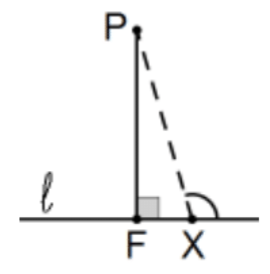
Corollary : The line of Prop 12 is unique. It is the line on the point that is perpendicular to the line.

Other immediate corollaries are Props 17 and 27. Some others are only a step away.

Proposition 17. In any triangle, two angles taken together in any manner are less than two right angles.

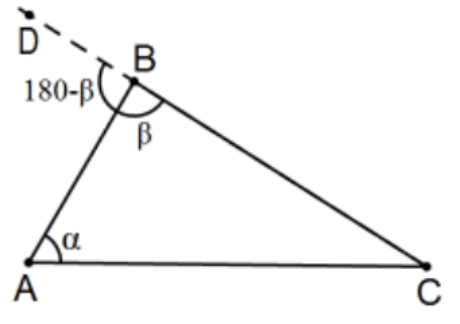
Proof : We focus on two of the angles, specifically those at A and B, of \(\triangle \mathrm{ABC}\) . By supplementary angles, \(\mathrm{m}(\angle \mathrm{CBA})+\mathrm{m}(\angle \mathrm{ABD})=\beta^{\circ}+(180-\beta)^{\circ}\) where \(\angle \mathrm{ABD}\) is a nonadjacent exterior angle to \(\angle \mathrm{A}\) so, by Prop. 16 , \(\mathrm{m}(\angle \mathrm{A})<\mathrm{m}(\angle \mathrm{ABD})\) and \(\alpha+\beta<\left(180^{\circ}-\beta\right)+\beta=180^{\circ}\) . Finally, \(\alpha+\beta<180^{\circ}\)

Proposition 18 . In any triangle, the greater side subtends the greater angle. [Angles opposite greater sides are greater. Note: Be careful which is which.]

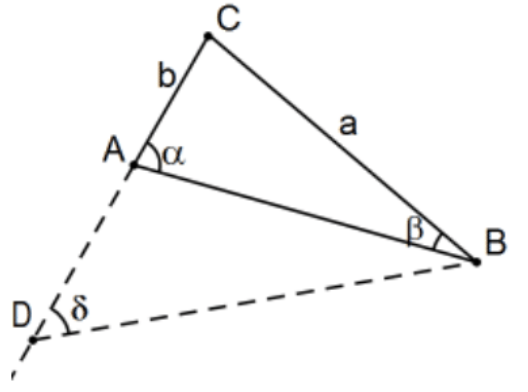
Proof 1 : In \(\triangle \mathrm{ABC}\) , assume \(a>b\) . Prove that \(\alpha>\beta\) .

Extend ray \(C A\) to point \(\mathrm{D}\) such that \(\mathrm{CD} \cong \mathrm{CB}\) . Then \(\angle \mathrm{D} \cong \angle \mathrm{CBD}\) (they are base angles of a triangle constructed to be isosceles) and point \(\mathrm{A}\) is in the interior of \(\angle C B D\) making \(\angle C A B\) an exterior angle wrt (with respect to) \(\angle \mathrm{ADB}\) of \(\triangle \mathrm{ADB}\) . By the Exterior Angle Theorem and the fact that \(\angle \mathrm{A}=\angle \mathrm{CAB}\) , we have that \(\delta<\alpha\) , and, since point \(\mathrm{A}\) is interior to \(\angle \mathrm{CBD}, \mathrm{m}(\angle \mathrm{B})(=\angle \mathrm{CBA})<\mathrm{m}(\angle \mathrm{CBD})\) . By transitivity, we have \(m(\angle B)<m(\angle A)\) or \(\alpha>\beta\) . QED.

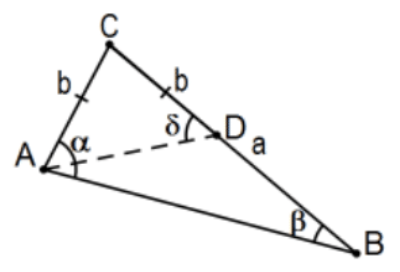
Proof 2 : As above, in \(\triangle \mathrm{ABC}\) , assume \(\mathrm{a}>\mathrm{b}\) . This time, let \(\mathrm{D}\) be along ray \(\mathrm{CB}\) such that \(\mathrm{CD} \cong \mathrm{CA}\) and proceed similarly. QED.

Proposition 19 . In any triangle, the greater angle subtends the greater side. [The converse of #18; i.e., sides opposite greater angles are greater. Again, if..., then...?]

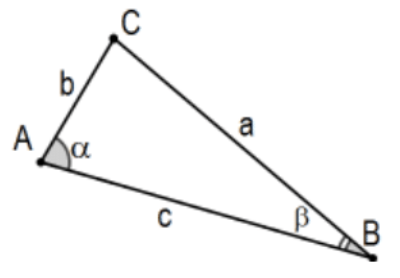
Proof : In \(\triangle \mathrm{ABC}\) pictured as in Prop.18 but assume that \(\angle \mathrm{A}>\angle \mathrm{B}\) . There are only three possibilities, \(a<b, a=b\) , or \(a>b\) . In the second case, the triangle would be isosceles and its base angles congruent, a contradiction to the hypothesis. In the first case, applying Prop 18, we would have that \(\angle \mathrm{A}<\angle \mathrm{B}\) ; again, a contradiction. Thus \(\mathrm{a}>\mathrm{b}\) . QED.

Note : Here, proof of the original statement made a proof of its converse to be very easy. Often that is the case (when the converse is true) but not necessarily. Sometimes a proof of the converse of statement can be quite different from using the validity of the original statement

Corollary : The shortest distance from a point to a line is the perpendicular distance.

Proof : Let \(F\) be the foot of the perpendicular on \(P\) and let \(X\) be any other point on the line. The greatest angle in the resulting triangle is... QED.

Proposition 20 [ Triangle Inequality .] In any triangle, two sides taken together in any manner are greater than the remaining one. In other words, the shortest distance between any two points is (surprise, surprise) the length of the line segment that joins them.

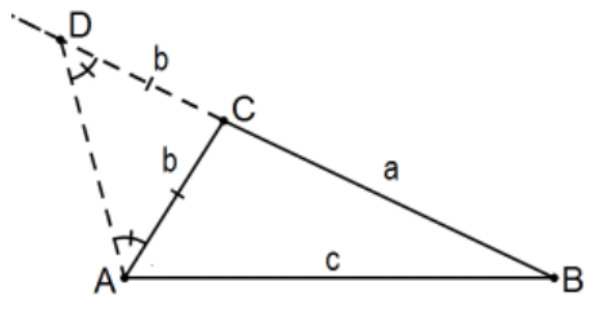
Proof : In the picture, we prove that \(a+b>c\) . Let \(D\) be the point along ray \(\mathrm{BC}\) of length \(\mathrm{b}\) making \(\triangle \mathrm{ACD}\) to be an isosceles triangle so that \(\angle \mathrm{D} \cong \angle \mathrm{DAC}\) . It is clear that \(\angle \mathrm{DAC}<\angle \mathrm{DAB}\) so that \(\angle \mathrm{ADB}<\angle \mathrm{DAB}\) . By Prop 19, AB \(<\mathrm{BC}+\mathrm{CD}\) . That is, \(a+b>c\) . QED.

Proposition 21 . If on one of the sides of a triangle, from its extremities, there be constructed two straight lines meeting within the triangle, the straight lines so constructed will be less than the remaining two sides of the triangle, but will contain a greater angle.

Note: The archaic language is a little hard to understand but the idea is quite simple. In any triangle \(\triangle \mathrm{ABC}\) , the triangle inequality says that the shortest distance between the two points A and C is the line segment that joins them. This proposition says that "going through" any interior point D of the triangle is shorter than following the sides of the triangle through B and that the angle determined is greater than the angle at that third point B.

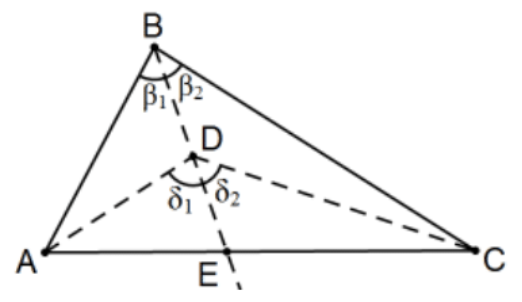
Proof : The angle situation is the easier of the two. Just consider ray \(\mathrm{BD}\) and use the EAT at \(\mathrm{D}\) by dividing the angle at \(\mathrm{B}\) into two angles of size \(\beta_{1}\) and \(\beta_{2}\) using ray BD that also divides \(\angle \mathrm{ADC}\) into two angles of size \(\delta_{1}\) and \(\delta_{2}\) with both \(\beta_{1}<\delta_{1}\) and \(\beta_{2}<\delta_{2}\) by the exterior angle theorem and adding, \(\beta=\beta_{1}+\beta_{2}<\delta_{1}+\delta_{2}=\delta\) so \(\angle \mathrm{ABC}<\angle \mathrm{ADC}\) . For the "shorter path" conclusion, extend ray CD to determine point \(\mathrm{E}\) as pictured. The result follows from careful use of the Triangle Inequality: \(\mathrm{AD}<\mathrm{AE}+\mathrm{ED}\) so that \(\mathrm{AD}+\mathrm{DC}<(\mathrm{AE}+\mathrm{ED})+\mathrm{DC}\) and reassociating, \(\mathrm{AD}+\mathrm{DC}<\mathrm{AE}+(\mathrm{ED}+\mathrm{DC})=\mathrm{AE}+\mathrm{EC}\) . But also \(\mathrm{EC}<\mathrm{EB}+\mathrm{BC}\) so that \(\mathrm{AE}+\mathrm{EC}<\mathrm{AE}+(\mathrm{EB}+\mathrm{BC})\) and, since \(\mathrm{AE}+\mathrm{EB}=\mathrm{AB}\) , we have that \(\mathrm{AD}+\mathrm{DC}<\mathrm{AB}+\mathrm{BC}\) .

Proposition 22. Out of three straight lines, which are equal to three given straight lines, to construct a triangle: thus it is necessary that two of the straight lines taken together in any manner should be greater than the remaining one. [The archaic language is awkward but the idea is simple.]

Proposition 23. Copying an angle using straightedge and compass was done immediately after Prop \(8.\)

Proposition 24 . If two triangles have the two sides equal to two sides respectively, but have the one of the angles contained by the equal straight lines greater than the other, they will also have the base greater than the base. [The Hinge Theorem , along with its converse Prop 25.]

Proof : In the figure, \(\mathrm{AB} \cong \mathrm{AB}^{\prime}\) and \(\angle \mathrm{BAC}<\angle \mathrm{B}^{\prime} \mathrm{AC}\) .

Prove \(\mathrm{BC}<\mathrm{B}^{\prime} \mathrm{C}\) , or more simply, \(\mathrm{x}+\mathrm{y}>\mathrm{z}\) .

Bisect \(\angle \mathrm{BAB}^{\prime}\) to determine point \(\mathrm{D}\) and \(\triangle \mathrm{BAD} \cong \triangle \mathrm{B}^{\prime} \mathrm{AD}\) by \(\mathrm{SAS}\) so \(\mathrm{BD} \cong \mathrm{B}^{\prime} \mathrm{D}\) . But \(\mathrm{BD}+\mathrm{DC}>\mathrm{BC}\) so we’re done. The picture could be misleading in that AD could shorter than \(\mathrm{AB}\) but the proof is the same. QED.

Proposition 25 . If two triangles have the two sides equal to two sides respectively, but have the base greater than the base, they will also have the one of the angles contained by the equal straight lines greater than the other. [The converse of Prop 24 and easily follows from it.]

Proposition 26 . If two triangles have the two angles equal to two angles respectively, and one side equal to one side, namely, either the side adjoining the equal angles, or that subtending one of the equal angles, they will also have the remaining sides equal to the remaining sides and the remaining angle to the remaining angle. [ Both ASA and AAS . These are different theorems with different proofs so a mild structural error!]

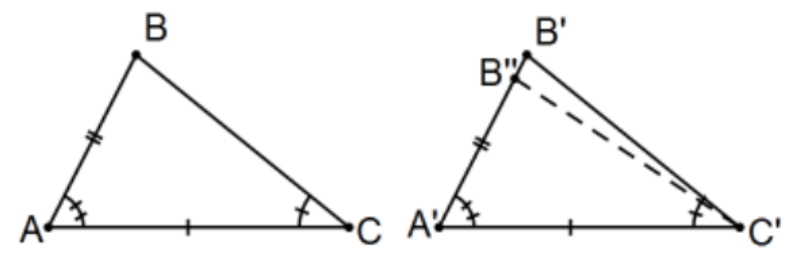
Proof of \(A S A:\) Assume \(\angle A \cong \angle \mathrm{A}^{\prime}, \angle \mathrm{C} \cong \angle \mathrm{C}^{\prime}\) , and \(\mathrm{AC} \cong \mathrm{A}^{\prime} \mathrm{C}^{\prime}\) . Let \(\mathrm{B}^{\prime \prime}\) be along ray \(\mathrm{A}^{\prime} \mathrm{B}^{\prime}\) such that \(\mathrm{AB} \cong \mathrm{A}^{\prime} \mathrm{B}^{\prime \prime}\) . Then \(\triangle \mathrm{ABC} \cong \triangle \mathrm{A}^{\prime} \mathrm{B}^{\prime \prime} \mathrm{C}^{\prime} \mathrm{by} \mathrm{SAS}\) so \(\angle C \cong \angle \mathrm{A}^{\prime} \mathrm{C}^{\prime} \mathrm{B}^{\prime \prime}\) by cpctc and, by transitivity, \(\angle \mathrm{C}^{\prime}=\angle \mathrm{A}^{\prime} \mathrm{C}^{\prime} \mathrm{B}^{\prime \prime}\) ; i.e., one and the same angle. Therefore, \(\mathrm{B}^{\prime}=\mathrm{B}^{\prime \prime}\) and the desired triangles are already known to be congruent. QED.

Proof of \(A A S: \quad\) Assume \(\angle \mathrm{A} \cong \angle \mathrm{A}^{\prime}, \angle \mathrm{C} \cong \angle \mathrm{C}^{\prime}\) , and \(A B \cong A^{\prime} B^{\prime}\) . Let \(C^{\prime \prime}\) be along ray \(A^{\prime} C^{\prime}\) such that \(\mathrm{AC} \cong \mathrm{A}^{\prime} \mathrm{C}^{\prime \prime}\) . Then \(\triangle \mathrm{ABC} \cong \triangle \mathrm{A}^{\prime} \mathrm{B}^{\prime} \mathrm{C}^{\prime \prime}\) by \(\mathrm{SAS}\) so \(\angle \mathrm{C} \cong \angle \mathrm{C}^{\prime \prime}\) by cpctc. If \(\mathrm{C}^{\prime} \neq \mathrm{C}^{\prime \prime}, \Delta \mathrm{B}^{\prime} \mathrm{C}^{\prime \prime} \mathrm{C}^{\prime}\) would be a non-degenerate triangle and would therefore violate the Exterior Angle Theorem. Since that is impossible, \(C^{\prime}=C^{\prime \prime}\) and, as before, the triangles are already known to be congruent. QED.

Proposition 27 . If a straight line falling on two straight lines make the alternate angles equal to one another, the straight lines will be parallel to one another. [With Prop 23, parallel lines exist!]

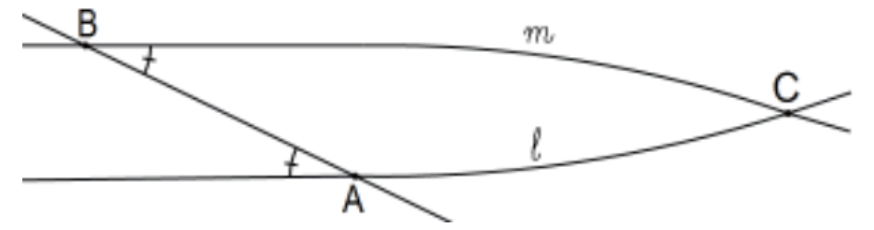
Proof : Suppose \(\angle \mathrm{A} \cong \angle \mathrm{B}\) but the lines \(\ell\) and \(m\) intersect at \(\mathrm{C}\) as pictured. Then \(\angle \mathrm{A}\) would be an exterior angle not adjacent to interior angle \(\angle \mathrm{B}\) with respect to \(\triangle \mathrm{ABC}\) . But by \(\mathrm{EAT}, \angle \mathrm{B}<\angle \mathrm{A}\) so that is impossible. That is, there is no such intersection on the right side. The lines can’t intersect on the other side either for the same reason by interchanging which angle is interior and which is exterior. QED.

Proposition 28 . If a straight line falling on two straight lines make the exterior angle equal to the interior and opposite angle on the same side, or the interior angles on the same side equal to two right angles, the straight lines will be parallel to one another. [Corollaries of Prop 27.]

Euclid’s Elements, Book 1, Prop 29 is the converse of Prop \(27-8\) and that would mean we’d be in Euclidean geometry since (along with the rest of the axioms we are assuming) it is logically equivalent to Euclid’s \(5^{\text {th }}\) Postulate and lots of variants some of which we’ll look at eventually. For now, we are focusing on neutral or, by another common name, absolute geometry. Although there are many later theorems in Euclid’s Elements (including in books that succeed Book 1) that are theorems of neutral geometry with identical proofs, the fact that Euclid went this far without using the \(5^{\text {th }}\) Postulate, including the limited form of the Exterior Angle Theorem, is persuasive indication that the Greeks were trying their best to prove it as a theorem instead of reluctantly accepting it as another axiom. For many centuries thereafter, very good geometers conducted extensive work in failed attempts to prove it. We will touch on a little of that in preliminary efforts of Saccheri and Lambert in the next chapter. Only the eventual acceptance of all of the other axioms along with the negation of Euclid’s \(5^{\text {th }}\) Postulate as a legitimate geometry in its own right, hyperbolic geometry, resolved the millennia-old problem now known as modern geometry.

If you're seeing this message, it means we're having trouble loading external resources on our website.

If you're behind a web filter, please make sure that the domains *.kastatic.org and *.kasandbox.org are unblocked.

To log in and use all the features of Khan Academy, please enable JavaScript in your browser.

Geometry (all content)

Unit 1: lines, unit 2: angles, unit 3: shapes, unit 4: triangles, unit 5: quadrilaterals, unit 6: coordinate plane, unit 7: area and perimeter, unit 8: volume and surface area, unit 9: pythagorean theorem, unit 10: transformations, unit 11: congruence, unit 12: similarity, unit 13: trigonometry, unit 14: circles, unit 15: analytic geometry, unit 16: geometric constructions, unit 17: miscellaneous.